Why can’t you divide by zero, even if you really want to?
- Tutorial
Recently on Habré an amazing article appeared, “Dad, why can’t you divide by zero?”, Which collected a lot of no less surprising comments.
Children's questions are usually very complex (“Why is the sky dark at night?”, “Why do apples fall on the ground?”) And adults usually do not have enough time to explain them clearly. And not always adults know the answer to these questions.
However, the question of division by zero is never one of the complex issues, and for me it remains a mystery why so many problems arise with it. Perhaps this is due to some flaws in the methodology of teaching mathematics in high school, in the difficulties of the transition from the study of arithmetic to the study of alphabetic algebra and the properties of elementary functions.
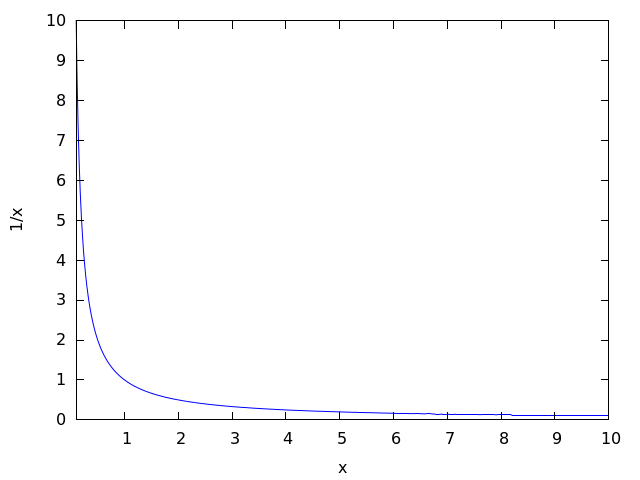
The most serious doubts arise, I think, after studying rational numbers, when for any number x, except zero, the concept of the inverse number 1 / x is introduced, and the graph of the hyperbola y (x) = 1 / x.
Obviously, when dividing 1 by very small numbers, very large numbers appear, and the smaller we take x, the more 1 / x becomes. Why can not we say that 1 / x = ∞ - there is some number?
The algebraic objection to this is as follows. Suppose that ∞ = 1 / x is a number. Then all rules should apply to this number.that have a place to be for ordinary numbers. In particular, on the one hand, the relation 0⋅∞ = 1 must be true, and on the other hand, since 0 = 1−1, 0⋅∞ = 1⋅∞ − 1⋅∞ = 0 must be satisfied. Thus, we have 1 = 0, and it already follows from this that all numbers are equal to each other and equal to zero. Indeed, since 1⋅x = x is true for any number x, then 1⋅x = 0⋅x = 0.
“Well, isn't that complete nonsense?” We ask ourselves when we get to this place.
Of course, this is complete nonsense if we are talking about ordinary numbers. But not without reason I emphasized the word "rules" above. We will return to them a little later, after considering the arithmetic objection to division by zero, and the beans will help us in this.
Let us return to those days when there were no computers, no calculators, or logarithmic rulers, and we set ourselves the task of dividing some random number, for example, by 5.
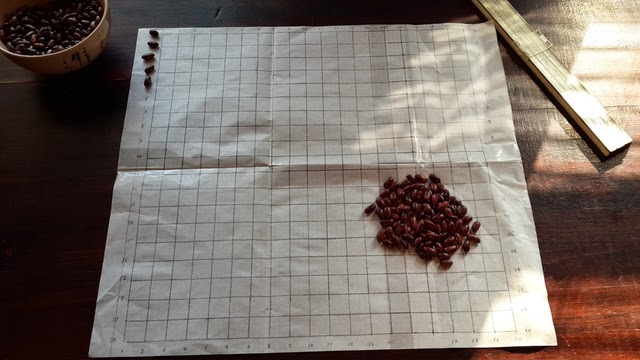
To do this, take a bowl with beans that symbolizes a natural row, and pour some from it then the number of grains per lined sheet of paper:

Thus, we set the dividend on our bean calculator.
The challenge is to arrange these grains in five rows. In order not to get confused, we mark these rows, that is, set the divider:
Now we lay out the grains from the heap into five rows in a column. This is much longer than on a conventional calculator, but it allows you to feel the beauty of arithmetic before the invention of the positional number system.

The algorithm ends when we get some rectangular number and (possibly) a remainder:

In this example, 2 grains remained, and rows of 5 grains formed 18. It turns out that the random number was 18⋅5 + 2 = 92.
It is clear that we can execute this algorithm for any natural divisible and any natural divisor other than zero; if the divisor is 0, then this algorithm is simply impossible to execute.
“Wait!” The attentive reader will say. - “In the considered example, we got the remainder 2, what to do with it?”
This is, in fact, a very important point. Generally speaking, we cannot divide beans without ruining our bean calculator - not only is it difficult to divide 2 beans into 5 identical parts, even if we split them appropriately, we will not be able to collect them.
Therefore, for a long time people tried to do without fractions. For example, in an anonymous 12th-century Arabic manuscript, the following task is described: "to share 100 pounds between 11 people." Since 100 = 11⋅9 + 1, the medieval mathematician suggests first distributing to each one 9 pounds, and then exchanging the remaining pound for eggs, which, as it turns out at the exchange rate, turns out to be exactly 91. But 91 = 11⋅8 + 3, therefore Arabic the scientist offers to distribute to each of 8 eggs, and give the three remaining eggs to the one who produces the section, or exchange it for salt to the eggs.
In modern mathematical language, the division was carried out in a semicircle of natural numbers. However, with the same success, using red and white beans, we could determine division with the remainder and in the ring of integers - in the described algorithm there would appear additional rules for choosing the colors used for calculating bean grains, but in the same way operations would remain senseless types x / 0 and 5/2.
Obviously, in order to give the 5/2 symbol a specific meaning, you need to change the rules of the game and go to the field of rational fractions, adding to the set of integers all kinds of expressions m / n, where m is an integer and n is a integer.
It is important to note that this can not be done in the only way, but in classical arithmetic we consider a completion in which the symbol 1 / n means the fraction of dividing 1 by n, i.e., a number for which the expression n⋅1 / n = 1; moreover, the shares do not make sense when counting piece objects (for example, bean grains), but when measuring quantities that are supposed to be continuous (or at least unlimitedly divisible) - lengths of segments, squares of figures, etc.
There is no sense in the field of rational fractions consider the partial quotient and the remainders, since the quotient of any nonzero divisor is some kind of rational fraction. Moreover, as in the case of natural numbers, we can use beans for dividing without changing the algorithm.
Indeed, let it be necessary to divide the rational number α = p / q by β = r / s. This is equivalent to performing the following actions:
α: β = p / q: r / s = p⋅s / q⋅r
and the problem for any rational α and β has been reduced to the already known procedure for dividing integers. This once again shows that division by zero has no arithmetic meaning.
“It turns out that you cannot divide by zero, even if you really want to?” - alas, the answer to this question is positive: we cannot determine the operation of division by zero based on their natural needs of counting and measurements. True, there are two loopholes.
First: instead of “ordinary” numbers (that is, rings of natural numbers and fields of rationals, as well as fields of real numbers, which, by the way, I still haven't said a word about and will tell you some other time), consider a degenerate case - trivial ring {0}, and put by definition 0/0 = 0. In this case, when we are told: “All numbers are equal to each other and equal to zero!” - we can say in a calm tone: “So what? It has always been like that. ”
Second: abandon some familiar rules of multiplication. In particular, from the axiom 0⋅x = 0. They say this is possible (see http://en.wikipedia.org/wiki/Wheel_theory ). Of course, this option is much more interesting than the first, but it also represents such a change in the rules of the game that immediately takes us beyond the framework of classical arithmetic.
In conclusion of this note I want to give a list of references for those who are interested in numerical systems:
- I.V. Arnold's “Theoretical Arithmetic”, M, OGIZ 1938 - a very detailed and detailed book in which one can find descriptions of classical numerical systems, including quaternions.
- EG Gonin “Theoretical Arithmetic”, M, 1959 — this book is shorter and more modern, and also very good, although not as detailed as the book of I.V. Arnold.
- S. Feferman "Numerical Systems" - a classic monograph, sometimes quite complicated; it sets out some particular questions that are not found in the other two books on theoretical arithmetic.
- A. A. Kirillov “What is a Number?” (1993) is a small brochure designed for a trained reader.
-E. B. Dynkin, V. A. Uspensky “Mathematical Conversations” is a popular book for schoolchildren. It contains a lot of information and tasks on such a "non-standard" topic as p-adic numbers.
Children's questions are usually very complex (“Why is the sky dark at night?”, “Why do apples fall on the ground?”) And adults usually do not have enough time to explain them clearly. And not always adults know the answer to these questions.
However, the question of division by zero is never one of the complex issues, and for me it remains a mystery why so many problems arise with it. Perhaps this is due to some flaws in the methodology of teaching mathematics in high school, in the difficulties of the transition from the study of arithmetic to the study of alphabetic algebra and the properties of elementary functions.

The most serious doubts arise, I think, after studying rational numbers, when for any number x, except zero, the concept of the inverse number 1 / x is introduced, and the graph of the hyperbola y (x) = 1 / x.
Obviously, when dividing 1 by very small numbers, very large numbers appear, and the smaller we take x, the more 1 / x becomes. Why can not we say that 1 / x = ∞ - there is some number?
The algebraic objection to this is as follows. Suppose that ∞ = 1 / x is a number. Then all rules should apply to this number.that have a place to be for ordinary numbers. In particular, on the one hand, the relation 0⋅∞ = 1 must be true, and on the other hand, since 0 = 1−1, 0⋅∞ = 1⋅∞ − 1⋅∞ = 0 must be satisfied. Thus, we have 1 = 0, and it already follows from this that all numbers are equal to each other and equal to zero. Indeed, since 1⋅x = x is true for any number x, then 1⋅x = 0⋅x = 0.
“Well, isn't that complete nonsense?” We ask ourselves when we get to this place.
Of course, this is complete nonsense if we are talking about ordinary numbers. But not without reason I emphasized the word "rules" above. We will return to them a little later, after considering the arithmetic objection to division by zero, and the beans will help us in this.
Let us return to those days when there were no computers, no calculators, or logarithmic rulers, and we set ourselves the task of dividing some random number, for example, by 5.
To do this, take a bowl with beans that symbolizes a natural row, and pour some from it then the number of grains per lined sheet of paper:

Thus, we set the dividend on our bean calculator.
The challenge is to arrange these grains in five rows. In order not to get confused, we mark these rows, that is, set the divider:

Now we lay out the grains from the heap into five rows in a column. This is much longer than on a conventional calculator, but it allows you to feel the beauty of arithmetic before the invention of the positional number system.

The algorithm ends when we get some rectangular number and (possibly) a remainder:

In this example, 2 grains remained, and rows of 5 grains formed 18. It turns out that the random number was 18⋅5 + 2 = 92.
It is clear that we can execute this algorithm for any natural divisible and any natural divisor other than zero; if the divisor is 0, then this algorithm is simply impossible to execute.
“Wait!” The attentive reader will say. - “In the considered example, we got the remainder 2, what to do with it?”
This is, in fact, a very important point. Generally speaking, we cannot divide beans without ruining our bean calculator - not only is it difficult to divide 2 beans into 5 identical parts, even if we split them appropriately, we will not be able to collect them.
Therefore, for a long time people tried to do without fractions. For example, in an anonymous 12th-century Arabic manuscript, the following task is described: "to share 100 pounds between 11 people." Since 100 = 11⋅9 + 1, the medieval mathematician suggests first distributing to each one 9 pounds, and then exchanging the remaining pound for eggs, which, as it turns out at the exchange rate, turns out to be exactly 91. But 91 = 11⋅8 + 3, therefore Arabic the scientist offers to distribute to each of 8 eggs, and give the three remaining eggs to the one who produces the section, or exchange it for salt to the eggs.
In modern mathematical language, the division was carried out in a semicircle of natural numbers. However, with the same success, using red and white beans, we could determine division with the remainder and in the ring of integers - in the described algorithm there would appear additional rules for choosing the colors used for calculating bean grains, but in the same way operations would remain senseless types x / 0 and 5/2.
Obviously, in order to give the 5/2 symbol a specific meaning, you need to change the rules of the game and go to the field of rational fractions, adding to the set of integers all kinds of expressions m / n, where m is an integer and n is a integer.
It is important to note that this can not be done in the only way, but in classical arithmetic we consider a completion in which the symbol 1 / n means the fraction of dividing 1 by n, i.e., a number for which the expression n⋅1 / n = 1; moreover, the shares do not make sense when counting piece objects (for example, bean grains), but when measuring quantities that are supposed to be continuous (or at least unlimitedly divisible) - lengths of segments, squares of figures, etc.
There is no sense in the field of rational fractions consider the partial quotient and the remainders, since the quotient of any nonzero divisor is some kind of rational fraction. Moreover, as in the case of natural numbers, we can use beans for dividing without changing the algorithm.
Indeed, let it be necessary to divide the rational number α = p / q by β = r / s. This is equivalent to performing the following actions:
α: β = p / q: r / s = p⋅s / q⋅r
and the problem for any rational α and β has been reduced to the already known procedure for dividing integers. This once again shows that division by zero has no arithmetic meaning.
“It turns out that you cannot divide by zero, even if you really want to?” - alas, the answer to this question is positive: we cannot determine the operation of division by zero based on their natural needs of counting and measurements. True, there are two loopholes.
First: instead of “ordinary” numbers (that is, rings of natural numbers and fields of rationals, as well as fields of real numbers, which, by the way, I still haven't said a word about and will tell you some other time), consider a degenerate case - trivial ring {0}, and put by definition 0/0 = 0. In this case, when we are told: “All numbers are equal to each other and equal to zero!” - we can say in a calm tone: “So what? It has always been like that. ”
Second: abandon some familiar rules of multiplication. In particular, from the axiom 0⋅x = 0. They say this is possible (see http://en.wikipedia.org/wiki/Wheel_theory ). Of course, this option is much more interesting than the first, but it also represents such a change in the rules of the game that immediately takes us beyond the framework of classical arithmetic.
In conclusion of this note I want to give a list of references for those who are interested in numerical systems:
- I.V. Arnold's “Theoretical Arithmetic”, M, OGIZ 1938 - a very detailed and detailed book in which one can find descriptions of classical numerical systems, including quaternions.
- EG Gonin “Theoretical Arithmetic”, M, 1959 — this book is shorter and more modern, and also very good, although not as detailed as the book of I.V. Arnold.
- S. Feferman "Numerical Systems" - a classic monograph, sometimes quite complicated; it sets out some particular questions that are not found in the other two books on theoretical arithmetic.
- A. A. Kirillov “What is a Number?” (1993) is a small brochure designed for a trained reader.
-E. B. Dynkin, V. A. Uspensky “Mathematical Conversations” is a popular book for schoolchildren. It contains a lot of information and tasks on such a "non-standard" topic as p-adic numbers.
