Math knitting
Strange attractor
Attractor (eng. Attract - attract, attract) is a compact subset of the phase space of a dynamical system, all trajectories from a certain neighborhood of which tend to it with time tending to infinity. One of the nominal examples of the attractor is the Lorenz attractor.
The Lorentz attractor was found in numerical experiments of Lorentz, who studied the behavior of trajectories of a nonlinear system:

for parameter values: σ = 10, r = 28, b = 8/3. The system arises in the following physical questions and models: convection in a closed loop, rotation of a water wheel, a single-mode laser model, and a dissipative harmonic oscillator. The Lorentz model is a real physical example of dynamical systems with chaotic behavior.

The mathematician Hinke Osing has a completely mathematical hobby - knitting. Counting loops in her free time, she rests. Once her supervisor, Professor Krauskopf imprudently threw: “Would you link something useful!”. And Dr. Osinga tied.
So there was a model of chaos. Now the world's only knitted chaos is spinning under the ceiling in the office of mathematicians at the University of Bristol.
Hinke Osinga, MD, University of Bristol: “I knitted every free minute. Mostly in the evenings. About 2 hours a day for almost two months. A total of 85 hours. 25 odd thousand loops, and chaos turned out. Moreover, very nice. " Now that you can touch it, it is easier for mathematicians to study it. They have been doing this for 2 years (2004 interview), modeling on a computer the “Lorentz equations”, which describe chaotic movements. Mathematicians promised a bottle of champagne to the first to offer another related model. Only two weeks later did the first letters with photos come.


Hyperbolic plane
Lobachevsky geometry (hyperbolic geometry) is one of non-Euclidean geometries, a geometric theory based on the same basic premises as ordinary Euclidean geometry, with the exception of the parallel axiom, which is replaced by the parallel Lobachevsky axiom. The following axiom is accepted in Lobachevsky’s geometry: at least two straight lines lying on the same line and not intersecting it pass through a point not lying on a given line.
The pseudosphere (Beltrami surface) is a surface of constant negative curvature formed by the rotation of the tractrix near its asymptote. The name emphasizes the similarities and differences with the sphere, which is an example of a surface with a curvature that is also constant but positive. The name “pseudosphere” of the surface was given by Beltrami.
He drew attention to the fact that the pseudosphere implements a local model of Lobachevsky geometry.

Daina Taimin solved the centennial problem of non-Euclidean geometry by visualizing hyperbolic planes. Hyperbolic planes are related to non-Euclidean geometry, which is traditionally difficult to visualize. Dayna Taimina managed to do this using knitted fabrics. She crocheted her first model of the hyperbolic plane in 1997 in order to use non-Euclidean geometry in the studio course. Since then, she has knitted more than a hundred geometric patterns.


Her technique is used in ecology. Margaret Wertheim leads the project to recreate the inhabitants of the coral reef, using the crochet (crochet) technique invented by the mathematician - glorifying the awesomeness of the coral reef, and plunging into the hyperbolic geometry that underlies the creation of coral.
Video on TED: Margaret Wertheim on the beautiful mathematics of coral (and crocheting) , which provides a simple explanation of Euclidean and hyperbolic space.
Klein Bottle
A Klein bottle is a non-orientable (one-sided) surface, first described in 1882 by the German mathematician F. Klein. It is closely connected with the Mobius strip and the projective plane. The name, apparently, comes from the incorrect translation of the German word Fläche (surface), which in German is close in spelling to the word Flasche (bottle); then this name returned in this form to German.
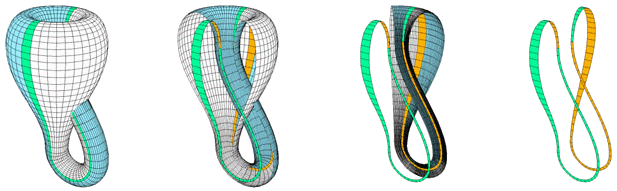
The Klein surface in the form of “figure 8”, shown in the figure below, can be represented as a system of equations with parameters, which looks much simpler than for a classic Klein bottle:




If you cut the Klein bottle into two halves along the plane of symmetry, you will get two mirror Mobius stripes, one with a half-turn to the right and the other with a half-turn to the left. In fact, it’s possible to dissect a Klein bottle so that one Mobius strip is obtained. Otherwise, a Klein bottle can be presented in the form of two Mobius bands connected to each other by a conventional double-sided tape. In the figure below, the inner surface of this tape is white and the outer blue.
Knitted Klein bottle:

As you can see, Acme also makes glass bottles.
Fractals
A fractal (lat. Fractus - crushed, broken, broken) is a mathematical set that has the property of self-similarity, that is, homogeneity in different measurement scales (any part of the fractal is similar to the whole set). In mathematics, fractals mean sets of points in Euclidean space that have a fractional metric dimension (in the sense of Minkowski or Hausdorff) or a metric dimension that is different from the topological one, therefore they should be distinguished from other geometric figures limited by a finite number of links.
Starting from the end of the 19th century, examples of self-similar objects with pathological properties from the point of view of classical analysis appear in mathematics. Sierpinski’s triangle is a fractal, one of the two-dimensional analogues of the Cantor set, proposed by the Polish mathematician Vaclav Sierpinski in 1915. Also known as the "wire rack" or "napkin" Sierpinski. The midpoints of the sides of the equilateral triangle T0 are connected by segments. It turns out 4 new triangles. The interior of the mid-triangle is removed from the original triangle. It turns out that the set T1 consists of the 3 remaining triangles of the “first rank”. By doing the same with each of the triangles of the first rank, we obtain the set T2 consisting of 9 equilateral triangles of the second rank. Continuing this process indefinitely, we obtain an infinite sequence T0⊃T1⊃ ⋯ ⊃Tn⊃ ...,

Dr. David Wilstrom, although a man, sometimes also knits. He was taught knitting at one of the textile workshops, and since then he has been doing interesting things from thread in his spare time.

And finally, a few more knitted fractals.
Pythagorean tree

These rugs were created by Woollythoughts. They also make unusual panels , the image on which is visible only at a certain angle.
Lots of Julia

Sources:
About the knitted attractor
Website of Hinke Osing and Bernd Krauskopf
Hyperbolic knitting
Daina Taimin
Hyperbolic knitting
About the exhibition Daina Taymini
Klein bottle
Acme klein bottle company
Mathematical Knitting Network
Pythagorean tree
Article on mathematical knitting, but images are not available
