What is the importance of 196 884 = 196 883 + 1? How to explain it on the fingers?
- Transfer
The author of the answer to Quora is Michael Griffin, a postdoc on mathematics
Senia Sheidwasser gave a very good, simple answer to this question, I recommend reading this short version. But there is a much more surprising story of the monstrous nonsense hypothesis (Monstrous Moonshine), mixed with Mackay's equation: from Jack Daniel's whiskey to black holes and quantum gravity.
Symmetries and mathematical “groups” are often mentioned in this story, so let's start with what is meant by a group in mathematics. A group can be presented as a way to reorder a set of objects, while maintaining a certain structure. Operations in a group must follow certain rules, for example, it must always be possible to cancel an operation, and if you perform one operation and then another, you will receive a third operationin a group .
Four variants of rotation and four axes of symmetry of the square. Image source
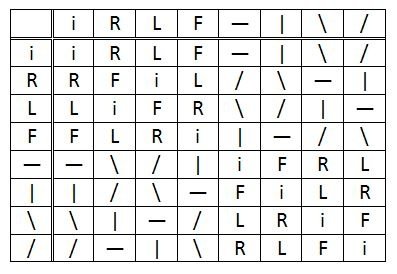
If you like to represent shapes, then a simple example of a group is the symmetry of a square. It can be rotated in three ways: 90 ° to the right (clockwise), 180 ° and 90 ° to the left (counterclockwise); There are four symmetries: vertical, horizontal, and two diagonal axes); and there is one symmetry of identitywhen nothing changes. If you rotate the square 90 ° to the right, and then reflect on the vertical axis, you get another symmetry. In particular, the result will be the same as if you immediately reflect on the diagonal axis from the upper left to the lower right corner. This is a kind of multiplication table for the elements of a group. In fact, we can write a multiplication table for a better understanding of the group structure. I did it right here. The symbol “i” in the table is the symmetry of an identity when nothing changes. “R” and “L” - rotate 90 ° to the right and left, respectively. “F” is a 180 ° rotation, and each line is a reflection along the axis in the direction of this line.
Some groups can be broken into smaller parts. For example, if you have two squares, then there may be two copies of the same symmetry operations, each of which acts on one square independently of the other. Simple groups cannot be broken up into smaller independent groups, so they are like primes in group theory. But finite simple groups are a little more difficult to classify than simple numbers. During the second half of the last century, significant progress was made in attempts to fully classify all finite simple groups. Most simple groups fit into neatly organized families. For example, one family contains all the symmetries of regular N-gons (such as an equilateral triangle, a square, a regular pentagon, etc.). But not all groups fit into any normal family. There are exactly 26 “sporadic” groups that are orphans. They are usually a bit more difficult to define, but many of them can be constructed from lattice symmetries in several dimensions. The largest of the simplest sporadic groups isMonster .
In 1973, Fisher and Griss first (independently) found evidence that a very large simple group can exist if it satisfies certain properties. But only a decade later, it was possible to prove that these properties are stable, and the group does exist. Griss called this elusive hypothetical group the Friendly Giant, the initials F. F. for Fisher-Griss. But Conway, a more famous mathematician, called her a Monster - and such a name stuck. By the way, this Conway plays an important role in our history, but most likely you have heard about it before. This is the same Conway who invented the game "Life" and proved the theorem of free will. If you do not remember, go read!
In 1975, two mathematicians, Ogg and Tits, met at a conference in Paris. Tits calculated that if the Monster exists, its size will be:
2 ^ 46 · 3 ^ 20 · 5 ^ 9 · 7 ^ 6 · 11 ^ 2 · 13 ^ 3 · 17 · 19 · 23 · 29 · 31 · 41 · 47 · 59 · 71
≈ 8 × 10 ^ 53
This is a very large number. Very, very, very big. This is the approximate number of atoms in Saturn and Jupiter combined. But Ogg’s attention did not attract size, but expansion into prime factors.
Ogg at that time was studying the pieces called modular curves. If N is a positive integer, then there is a surface, let's call it X (N), which captures some important arithmetic information about the number N (if you remember from school complex numbers, then such a surface can be obtained by “rolling” or “folding” complex a plane with a series of symmetries, depending on the number N). Ogg asked a question like this: if N is a prime number, then in what case will this surface (or modular curve) look like a ball, and not a donut with one or more handles (that is, “holes” in the donut)? He found that only if N belongs to the set
{2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 41, 47, 59, 71}
These are the same primes that are used in the calculation of Tits for the size of the Monster! But between these two calculations there is absolutely no obvious connection. Ogg was so stunned by this apparent coincidence that he offered a bottle of Jack Daniel's whiskey to anyone who could explain it.
For obvious reasons, drawing up the multiplication table does not help to study the Monster. If we write down the multiplication table with hydrogen atoms, it will not fit in our galaxy. Instead, mathematicians managed to create a table of Monster symbols.. Yes, it sounds like a guide to the game Dungeons & Dragons, and maybe this is not the worst way to present a table. This is a kind of Necronomicon for Monster; the table of numbers 194 × 194, giving mathematicians some deep understanding of the astronomically huge Monster. The first column lists the “size of irreducible representations” of the Monster. These are bizarre words, but the essence of our history is that the first two values in the first column are the numbers 1 and 196 883 . This is where McKay's equation appears.
Mackay famously pointed out to Conway that
196884 = 1 + 196883
Conway considered McKay's hypothesis so ridiculous that he called it fantasy or nonsense (moonshine). In this equation, 196884 is the firstcoefficient of an important function, called the J-function , which mathematicians have been studying for a very long time. Here we again begin to return to Ogg and his question on a bottle of "Jack Daniels."
The J-function is a modular function, that is, it takes a point with a modular curve, like those that Ogg studied - and gives a number (again, if you are familiar with complex numbers, you can think of a modular function as a function on ordinary complex numbers, but with an obscene amount of symmetry). It is difficult to more clearly explain what a modular function is, but do not dwell on it.
Image source
In addition, the J-function is the most basic modular function for the simplest modular curve X (1). This is the most “basic” function in the sense that any other modular function for X (1) can be written as a polynomial or a polynomial relation in a J-function. Some other modular curves, such as X (2), have another basic modular function. Call it J_2. In fact, for X (N), the basic modular function J_N of this kind is precisely when the form X (N) is a ball (without “handles” or “holes”), exactly as Ogg studied.
Another mathematician Thompson realized that the observation of Mackay can be developed. He noted that the following several coefficients of the original J-function can also be written as sums of values from the first column of the Monster symbol table. Moreover, you can write several coefficients of other functions J_N as sums of other values from the table. At that time, Thompson was still working with an incomplete symbol table. It was only in 1979 that Fisher, Livingston and Thorn completed the calculation of the symbol table, and later that year Conway and Norton turned Thompson’s observations into an exact hypothesis. They argued that there is a way to write any coefficient of the J-function as the sum of the dimensions of the irreducible Monster representations (that is, the entries from the first column of the Monster symbol table). Moreover, it can be done in such a way that if we swap records from the first column with records from another column of the symbol table, we get the coefficients of one of the other functions J_N! For example, here are the first three coefficients of the original J-function (on the left side of the equations):
196884 = 1 + 196883,
21493760 = 1 + 196883 + 21296876, and
864299970 = 2 × 1 + 2 × 196883 + 21296876 + 842609326,
where 1 , 196883 , 21296876 , and 842609326 are the first four values in the first column of the Monster symbol table. And here are the first three coefficients of the function J_2 (again, on the left side of the equations):
4372 = 1 + 4371
96256 = 1 + 4371 + 91884 and
1240002 = 2 × 1 + 2 × 4371 + 91884 + 1139374,
where 1 , 4371 , 91884 and 1139374 - the first four values in the secondMonster symbol table column. And so on: each column of the symbol table gives the coefficients of the base modular function for some modular curves. Conway and Norton called their hypothesis monstrous nonsense (Monstrous Moonshine).
About a year ago I had a chance to talk with Conway about how this hypothesis appeared. He said that he was looking at the latest values in the Monster symbol table, which had taken so much effort to calculate, and then went down to the math library and opened the book, written decades ago, with tables of coefficients of modular functions. And he described this feeling of deep horror when the same numbers or their obvious combinations looked at him from the pages of an old book.
In 1982, Griss finally showed how to build a Monster. For the first time, mathematicians were able to get rid of the clause "if the Monster exists." Ten years later, Borchers, a former student of Conway, proved the hypothesis using the theory of “vertex operator algebras,” which he created specifically for this purpose. This theory was created on the basis of the old physical theory of the 1960s. Borchers received the 1998 Fields Medal, largely for this proof. This is a kind of Nobel Prize in mathematics, except that for some inexplicable reason you need to be under 40 years old to get it. As I heard, Ogg satisfied Borchers' answer to his question, but Borchers does not drink, so the bottle of “Jack Daniels” remains unclaimed. On the other hand, although Conway is very pleased with the work of Borchers, but he still sees in it only a check, but not an explanation.
The story does not end there. In 2007, Witten worked on conflict resolution in quantum gravity. Quantum mechanics and general relativity are not very compatible. Witten worked on a simplified question, discarding everything from the theory of relativity except gravity. He found reason to believe that the VOA from the hypothesis is the key to the theory of gravity in this simplified construction. In this theory, the J-function becomes a partition function, which counts various energy states. Here various Monster symbols appear that correspond to the states of the black hole. Witten asked if some of these black hole states are more common than others. Returning back to Monster, it basically comes down to the question of how many unitsdo we expect to see when we split a given J-factor? Or how many times will fall 196 883 ? Are units rare? Or are there basically units with several interesting meanings scattered here and there? I think many people have this question when they first encounter the hypothesis of monstrous nonsense. If everything came down to units , it would make the theory much less interesting. But do not worry about it. Despite the fact that from the very beginning we meet a few, they become very rare when we go to larger coefficients, and larger characters begin to take over. After the 200th coefficient, the symbols mostly appear in proportion to the size of their measurement. The ratio of 1 with all other characters is about 1 to 5.8 × 10 ^ 27. This is roughly the ratio of the mass of the clip and the mass of the Earth. The second character in size is found 196883 times more often, the third - 21296876 times more often, etc. Returning to the Witten configuration, this means that the larger energy states for the black hole are more common, while the trivial vacuum state ( 1 ) practically does not exist.
There are many more studies on this topic. We (mathematicians) observed (and in some cases proved) a phenomenon for other groups outside the Monster. String theory specialists continue to pry into our work, hoping to turn these new variations into new theories of gravity.
For more tech-savvy readers who are interested in the details, I recommend the book "Nonsense Outside the Monster" by Terry Gannon or this scientific article (in open access).
Senia Sheidwasser gave a very good, simple answer to this question, I recommend reading this short version. But there is a much more surprising story of the monstrous nonsense hypothesis (Monstrous Moonshine), mixed with Mackay's equation: from Jack Daniel's whiskey to black holes and quantum gravity. Symmetries and mathematical “groups” are often mentioned in this story, so let's start with what is meant by a group in mathematics. A group can be presented as a way to reorder a set of objects, while maintaining a certain structure. Operations in a group must follow certain rules, for example, it must always be possible to cancel an operation, and if you perform one operation and then another, you will receive a third operationin a group .
Four variants of rotation and four axes of symmetry of the square. Image source
If you like to represent shapes, then a simple example of a group is the symmetry of a square. It can be rotated in three ways: 90 ° to the right (clockwise), 180 ° and 90 ° to the left (counterclockwise); There are four symmetries: vertical, horizontal, and two diagonal axes); and there is one symmetry of identitywhen nothing changes. If you rotate the square 90 ° to the right, and then reflect on the vertical axis, you get another symmetry. In particular, the result will be the same as if you immediately reflect on the diagonal axis from the upper left to the lower right corner. This is a kind of multiplication table for the elements of a group. In fact, we can write a multiplication table for a better understanding of the group structure. I did it right here. The symbol “i” in the table is the symmetry of an identity when nothing changes. “R” and “L” - rotate 90 ° to the right and left, respectively. “F” is a 180 ° rotation, and each line is a reflection along the axis in the direction of this line.
Some groups can be broken into smaller parts. For example, if you have two squares, then there may be two copies of the same symmetry operations, each of which acts on one square independently of the other. Simple groups cannot be broken up into smaller independent groups, so they are like primes in group theory. But finite simple groups are a little more difficult to classify than simple numbers. During the second half of the last century, significant progress was made in attempts to fully classify all finite simple groups. Most simple groups fit into neatly organized families. For example, one family contains all the symmetries of regular N-gons (such as an equilateral triangle, a square, a regular pentagon, etc.). But not all groups fit into any normal family. There are exactly 26 “sporadic” groups that are orphans. They are usually a bit more difficult to define, but many of them can be constructed from lattice symmetries in several dimensions. The largest of the simplest sporadic groups isMonster .
In 1973, Fisher and Griss first (independently) found evidence that a very large simple group can exist if it satisfies certain properties. But only a decade later, it was possible to prove that these properties are stable, and the group does exist. Griss called this elusive hypothetical group the Friendly Giant, the initials F. F. for Fisher-Griss. But Conway, a more famous mathematician, called her a Monster - and such a name stuck. By the way, this Conway plays an important role in our history, but most likely you have heard about it before. This is the same Conway who invented the game "Life" and proved the theorem of free will. If you do not remember, go read!
In 1975, two mathematicians, Ogg and Tits, met at a conference in Paris. Tits calculated that if the Monster exists, its size will be:
2 ^ 46 · 3 ^ 20 · 5 ^ 9 · 7 ^ 6 · 11 ^ 2 · 13 ^ 3 · 17 · 19 · 23 · 29 · 31 · 41 · 47 · 59 · 71
≈ 8 × 10 ^ 53
This is a very large number. Very, very, very big. This is the approximate number of atoms in Saturn and Jupiter combined. But Ogg’s attention did not attract size, but expansion into prime factors.
Ogg at that time was studying the pieces called modular curves. If N is a positive integer, then there is a surface, let's call it X (N), which captures some important arithmetic information about the number N (if you remember from school complex numbers, then such a surface can be obtained by “rolling” or “folding” complex a plane with a series of symmetries, depending on the number N). Ogg asked a question like this: if N is a prime number, then in what case will this surface (or modular curve) look like a ball, and not a donut with one or more handles (that is, “holes” in the donut)? He found that only if N belongs to the set
{2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 41, 47, 59, 71}
These are the same primes that are used in the calculation of Tits for the size of the Monster! But between these two calculations there is absolutely no obvious connection. Ogg was so stunned by this apparent coincidence that he offered a bottle of Jack Daniel's whiskey to anyone who could explain it.
For obvious reasons, drawing up the multiplication table does not help to study the Monster. If we write down the multiplication table with hydrogen atoms, it will not fit in our galaxy. Instead, mathematicians managed to create a table of Monster symbols.. Yes, it sounds like a guide to the game Dungeons & Dragons, and maybe this is not the worst way to present a table. This is a kind of Necronomicon for Monster; the table of numbers 194 × 194, giving mathematicians some deep understanding of the astronomically huge Monster. The first column lists the “size of irreducible representations” of the Monster. These are bizarre words, but the essence of our history is that the first two values in the first column are the numbers 1 and 196 883 . This is where McKay's equation appears.
Mackay famously pointed out to Conway that
196884 = 1 + 196883
Conway considered McKay's hypothesis so ridiculous that he called it fantasy or nonsense (moonshine). In this equation, 196884 is the firstcoefficient of an important function, called the J-function , which mathematicians have been studying for a very long time. Here we again begin to return to Ogg and his question on a bottle of "Jack Daniels."
The J-function is a modular function, that is, it takes a point with a modular curve, like those that Ogg studied - and gives a number (again, if you are familiar with complex numbers, you can think of a modular function as a function on ordinary complex numbers, but with an obscene amount of symmetry). It is difficult to more clearly explain what a modular function is, but do not dwell on it.
Image source
In addition, the J-function is the most basic modular function for the simplest modular curve X (1). This is the most “basic” function in the sense that any other modular function for X (1) can be written as a polynomial or a polynomial relation in a J-function. Some other modular curves, such as X (2), have another basic modular function. Call it J_2. In fact, for X (N), the basic modular function J_N of this kind is precisely when the form X (N) is a ball (without “handles” or “holes”), exactly as Ogg studied.
Another mathematician Thompson realized that the observation of Mackay can be developed. He noted that the following several coefficients of the original J-function can also be written as sums of values from the first column of the Monster symbol table. Moreover, you can write several coefficients of other functions J_N as sums of other values from the table. At that time, Thompson was still working with an incomplete symbol table. It was only in 1979 that Fisher, Livingston and Thorn completed the calculation of the symbol table, and later that year Conway and Norton turned Thompson’s observations into an exact hypothesis. They argued that there is a way to write any coefficient of the J-function as the sum of the dimensions of the irreducible Monster representations (that is, the entries from the first column of the Monster symbol table). Moreover, it can be done in such a way that if we swap records from the first column with records from another column of the symbol table, we get the coefficients of one of the other functions J_N! For example, here are the first three coefficients of the original J-function (on the left side of the equations):
196884 = 1 + 196883,
21493760 = 1 + 196883 + 21296876, and
864299970 = 2 × 1 + 2 × 196883 + 21296876 + 842609326,
where 1 , 196883 , 21296876 , and 842609326 are the first four values in the first column of the Monster symbol table. And here are the first three coefficients of the function J_2 (again, on the left side of the equations):
4372 = 1 + 4371
96256 = 1 + 4371 + 91884 and
1240002 = 2 × 1 + 2 × 4371 + 91884 + 1139374,
where 1 , 4371 , 91884 and 1139374 - the first four values in the secondMonster symbol table column. And so on: each column of the symbol table gives the coefficients of the base modular function for some modular curves. Conway and Norton called their hypothesis monstrous nonsense (Monstrous Moonshine).
About a year ago I had a chance to talk with Conway about how this hypothesis appeared. He said that he was looking at the latest values in the Monster symbol table, which had taken so much effort to calculate, and then went down to the math library and opened the book, written decades ago, with tables of coefficients of modular functions. And he described this feeling of deep horror when the same numbers or their obvious combinations looked at him from the pages of an old book.
In 1982, Griss finally showed how to build a Monster. For the first time, mathematicians were able to get rid of the clause "if the Monster exists." Ten years later, Borchers, a former student of Conway, proved the hypothesis using the theory of “vertex operator algebras,” which he created specifically for this purpose. This theory was created on the basis of the old physical theory of the 1960s. Borchers received the 1998 Fields Medal, largely for this proof. This is a kind of Nobel Prize in mathematics, except that for some inexplicable reason you need to be under 40 years old to get it. As I heard, Ogg satisfied Borchers' answer to his question, but Borchers does not drink, so the bottle of “Jack Daniels” remains unclaimed. On the other hand, although Conway is very pleased with the work of Borchers, but he still sees in it only a check, but not an explanation.
The story does not end there. In 2007, Witten worked on conflict resolution in quantum gravity. Quantum mechanics and general relativity are not very compatible. Witten worked on a simplified question, discarding everything from the theory of relativity except gravity. He found reason to believe that the VOA from the hypothesis is the key to the theory of gravity in this simplified construction. In this theory, the J-function becomes a partition function, which counts various energy states. Here various Monster symbols appear that correspond to the states of the black hole. Witten asked if some of these black hole states are more common than others. Returning back to Monster, it basically comes down to the question of how many unitsdo we expect to see when we split a given J-factor? Or how many times will fall 196 883 ? Are units rare? Or are there basically units with several interesting meanings scattered here and there? I think many people have this question when they first encounter the hypothesis of monstrous nonsense. If everything came down to units , it would make the theory much less interesting. But do not worry about it. Despite the fact that from the very beginning we meet a few, they become very rare when we go to larger coefficients, and larger characters begin to take over. After the 200th coefficient, the symbols mostly appear in proportion to the size of their measurement. The ratio of 1 with all other characters is about 1 to 5.8 × 10 ^ 27. This is roughly the ratio of the mass of the clip and the mass of the Earth. The second character in size is found 196883 times more often, the third - 21296876 times more often, etc. Returning to the Witten configuration, this means that the larger energy states for the black hole are more common, while the trivial vacuum state ( 1 ) practically does not exist.
There are many more studies on this topic. We (mathematicians) observed (and in some cases proved) a phenomenon for other groups outside the Monster. String theory specialists continue to pry into our work, hoping to turn these new variations into new theories of gravity.
For more tech-savvy readers who are interested in the details, I recommend the book "Nonsense Outside the Monster" by Terry Gannon or this scientific article (in open access).