Changing the capacitance of ceramic capacitors from temperature and voltage, or how your 4.7μF capacitor turns into 0.33μF

Introduction: I was puzzled.

A few years ago, after more than 25 years of working with these things, I learned something new about ceramic capacitors. While working on the LED lamp driver, I found that the time constant of the RC chain in my circuit does not look much like the calculated one.

Assuming that the wrong components were soldered to the board, I measured the resistance of the two resistors making up the voltage divider - they were very accurate. Then the capacitor was soldered - it was also magnificent. Just to make sure, I took new resistors and a capacitor, measured them, and soldered back. After that, I turned on the circuit, checked the main indicators, and expected to see that my problem with the RC chain was solved ... If only.

I tested the circuit in its natural environment: in the casing, which in turn was sheathed by itself to simulate the casing of a ceiling lamp. The temperature of the components in some places reached more than 100ºC. To be sure, and to refresh my memory, I re-read the datasheet on the used capacitors. Thus began my rethinking of ceramic capacitors.

Reference information on the main types of ceramic capacitors.

For those who do not remember this (like almost everyone), table 1 shows the labeling of the main types of capacitors and its meaning. This table describes the capacitors of the second and third class . Without going deep into details, first-class capacitors are usually made on a dielectric such as C0G (NP0).

Table 1.

Lower operating temperature		Upper working temperature		Change capacity in range (max.)
Symbol	Temperature (ºC)	Symbol	Temperature (ºC)	Symbol	Change (%)
Z	+10	2	+45	A	± 1.0
Y	-thirty	4	+65	B	± 1.5
X	-55	5	+85	C	± 2.2
-	-	6	+105	D	± 3.3
-	-	7	+125	E	± 4.7
-	-	8	+150	F	± 7.5
-	-	9	+200	P	± 10
-	-	-	-	R	± 15
-	-	-	-	S	± 22
-	-	-	-	T	+22, -33
-	-	-	-	U	+22, -56
-	-	-	-	V	+22, -82

Of the above on my life path, I most often came across capacitors such as X5R, X7R and Y5V. I never used capacitors like Y5V because of their extremely high sensitivity to external influences.

When a capacitor manufacturer develops a new product, he selects the dielectric so that the capacitance of the capacitor changes no more than certain limits in a certain temperature range. The X7R capacitors that I use should not change their capacity by more than ± 15% (third character) when the temperature changes from -55ºC (first character) to + 125ºC (second character). So either I got a bad game, or something else happens in my scheme.

Not all X7Rs are created the same.

Since the change in the time constant of my RC chain was much larger than could be explained by the temperature coefficient of capacity, I had to dig deeper. Looking at how much the capacitance of my capacitor swam away from the voltage applied to it, I was very surprised. The result was very far from the face value that was soldered. I took a 16V capacitor to work in a 12V circuit. Datashit said that my 4.7 microfarads convert to 1.5 microfarads under such conditions. This explained my problem.

Datashit also said that if you only increase the size from 0805 to 1206, then the resulting capacitance under the same conditions will already be 3.4 microfarads! This moment required a closer look.

I found that the Murata® and TDK® sites have cool tools for plotting capacitor capacitance versus various conditions. I drove 4.7 microfarad ceramic capacitors through them for different sizes and rated voltages. On Figure 1 shows graphs constructed Murata. Capacitors X5R and X7R were taken in sizes from 0603 to 1812 for voltage from 6.3 to 25V.

Figure 1. Change in capacitance depending on the applied voltage for the selected capacitors.


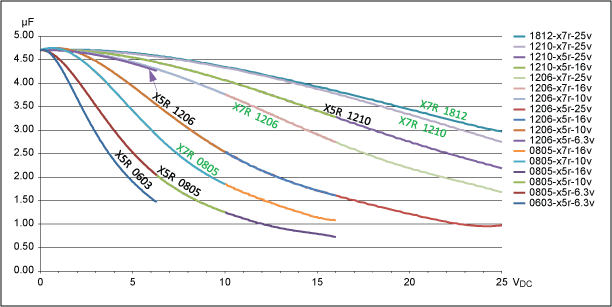
Please note that firstly, with an increase in size, the change in capacity decreases depending on the applied voltage, and vice versa.

The second interesting point is that, unlike the type of dielectric and size, the rated voltage does not seem to affect anything. I would expect a 25V capacitor at 12V to change its capacity less than a 16V capacitor at the same voltage. Looking at the graph for size 1206 X5R, we see that the 6.3V capacitor actually behaves better than its relatives at a higher rated voltage.

If we take a wider range of capacitors, we will see that this behavior is characteristic of all ceramic capacitors as a whole.

The third observation is that the X7R with the same size has less sensitivity to voltage changes than the X5R. I do not know how universal this rule is, but in my case it is.

Using the data of the graphs, we maketable 2 , showing how much the capacitance of X7R capacitors decreases at 12V.

Table 2. Reducing the capacitance of X7R capacitors of different sizes at a voltage of 12V.

Size	Capacitance, microfarad	% of face value
0805	1,53	32.6
1206	3.43	73.0
1210	4.16	88.5
1812	4.18	88.9
Face value	4.7	100

We see a steady improvement in the situation as the size of the case increases until we reach size 1210. A further increase in the case no longer makes sense.

In my case, I chose the smallest possible component size, since this parameter was critical for my project. In my ignorance, I believed that any X7R capacitor would work just as well as another with the same dielectric - and was wrong. For the RC-chain to work correctly, I had to take a capacitor of the same rating, but in a larger case.

Choosing the right capacitor

I really did not want to use a capacitor of size 1210. Fortunately, I had the opportunity to increase the resistance of the resistors by five times, while reducing the capacitance to 1uF. The graphs in Figure 2 show the behavior of various X7R capacitors of 1 μF at 16V compared to their counterparts X7R 4.7 μF at 16V.

Figure 2. The behavior of various capacitors at 1uF and 4.7uF.


Capacitor 0603 1uF behaves the same as 0805 4.7uF. Taken together, 0805 and 1206 per 1 microfarad feel better than 4.7 microfarads of frame size 1210. Using a 1 microfarad capacitor in the 0805 package, I could maintain the requirements for component sizes, while at the same time I received 85% of the initial capacitance, and not 30%, as was earlier.

But that is not all. I was pretty puzzled, for I thought that all X7R capacitorsshould have similar coefficients of change of capacitance from voltage, since all are made on the same dielectric - namely, X7R. I contacted a colleague - a specialist in ceramic capacitors ¹ . He explained that there are many materials that qualify as “X7R”. In fact, any material that allows the component to function in the temperature range from -55ºC to + 125ºC with a change in characteristics of no more than ± 15% can be called “X7R”. He also said that there are no specifications for the coefficient of change of capacitance from voltage for either the X7R or any other types.

This is a very important point, and I will repeat it. The manufacturer may call the capacitor X7R (or X5R, or something else) as long as it meets the tolerances for the temperature coefficient of capacity. Regardless of how bad its voltage coefficient is.

For a development engineer, this fact only refreshes the old joke - “any experienced engineer knows: read the datasheet!”

Manufacturers are releasing increasingly smaller components, and are forced to seek compromise materials. In order to provide the necessary capacitive-dimensional indicators, they have to degrade the voltage coefficients. Of course, more reputable manufacturers are doing everything possible to minimize the adverse effects of this compromise.

What about the type Y5V that I immediately dropped? For a check in the head, let's look at a conventional Y5V capacitor. I will not highlight any specific manufacturer of these capacitors - all are about the same. We’ll select 4.7 microfarads at 6.3V in the 0603 package, and see its parameters at a temperature of + 85ºC and a voltage of 5V. Typical capacity is 92.3% lower than nominal, or 0.33uF. This is true. By attaching 5V to this capacitor, we get a drop in capacitance of 14 times compared to the nominal.

At a temperature of + 85ºC and a voltage of 0V, the capacitance decreases by 68.14%, from 4.7 μF to 1.5 μF. It can be assumed that by applying 5V we get a further decrease in capacitance - from 0.33 μF to 0.11 μF. Fortunately, these effects are not combined. A decrease in capacitance at 5V at room temperature is much worse than at + 85ºC.

For clarity, in this case, at a voltage of 0 V, the capacitance drops from 4.7 μF to 1.5 μF at + 85ºC, while at a voltage of 5 V, the capacitance increases from 0.33 μF at room temperature, to 0.39 μF at + 85 ° C. This should convince you to really carefully check all the specifications of the components that you use.

Conclusion

As a result of this tutorial, I no longer just point out the X7R or X5R types to colleagues or suppliers. Instead, I indicate specific batches of specific suppliers that I myself checked. I also warn customers to double-check specifications when considering alternative suppliers for production to ensure that they do not run into these problems.

The main conclusion from this whole story, as you probably guessed, is: “read datasheets!”. Is always. With no exceptions. Request more data if the datasheet does not contain enough information. Remember that the designations of ceramic capacitors are X7V, Y5V, etc. say absolutely nothing about their voltage coefficients. Engineers need to double-check the data to know, to really know how the capacitors used will behave in real conditions. All in all, keep in mind in our crazy race for smaller and smaller dimensions this is becoming an increasingly important moment every day.

about the author

Mark Fortunato spent most of his life trying to get these nasty electrons to be in the right place at the right time. He worked on various things - from speech recognition systems and microwave equipment, to LED lamps (those that are regulated correctly, mind you!). He spent the past 16 years helping customers tame their analog circuits. Mr. Fortunato is now a leading specialist at Maxim Integrated Communications and Automotive Solutions. When he does not graze electrons, Mark loves to train young people, read journalism, watch his youngest son play lacrosse, and the eldest son plays music. In general, he seeks to live in harmony. Mark is very sorry that he will no longer meet with Jim Williams or Bob Pease.

Footnotes

¹ The author would like to thank Chris Burkett, application engineers from TDK for its explanation "that is, hell is going on."

Murata is a registered trademark of Murata Manufacturing Co., Ltd.

TDK is a registered service mark and registered trademark of TDK Corporation.

---

PS At the request of workers - a comparative photo of capacitors of various sizes. 5mm mesh pitch.