About the diagonal of a square
The introduction of complex numbers into circulation was far from the first revolution in man's understanding of the nature of number. Two thousand years before this, the world of ancient Greek mathematics experienced a tremendous shock.
The troubles among the Pythagoreans did not begin immediately. The scientific school founded by Pythagoras ended up badly, but today's story is not about the vigorous pogrom that was inflicted on the Pythagoreans by the people thankful for enlightenment, but more about spiritual ups and downs.
The term "scientific school" in relation to the organization founded by Pythagoras is a kind of euphemism. Taking a healthy look at its structure and applied technologies, Pythagoreanism should be safely attributed to totalitarian cults, which was quite in the spirit of the times (however, it is always in the spirit of the times, an eternal classic). There was a separation into degrees of initiation, and a complex system of rituals with prohibitions (for example, well-known, such as “do not eat beans” or “do not bite off a whole bun”), and a complex philosophical creed. Hello to Ron Hubbard and his comrades. There is nothing new under the sun.
In general, during the life of Pythagoras, his “school” was a solid enterprise, besides having significant and ever-growing political influence.
In general, the philosophy of Pythagoras had a significant impact on Western culture (including us). Many ideas found their development in classical Greek philosophy, and already everyone knows about the Pythagorean theorem. The expression "harmony of the spheres", by the way, also dates back to the Pythagoreans.
One of the essential elements of their philosophy was the idea that any number can be represented as the ratio of two integers, that is, in the form of a simple fraction. In this they, in particular, saw the perfection of the nature of number. Moreover, it seemed quite obvious. In modern mathematics, such numbers are called rational, and their number is indicated by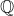 . Now pause for a few seconds, think, where does it generally follow that this is not so? Can you give an argument that would be convincing enough for the ancient Greek? Well, or at least convincing enough for yourself personally?
. Now pause for a few seconds, think, where does it generally follow that this is not so? Can you give an argument that would be convincing enough for the ancient Greek? Well, or at least convincing enough for yourself personally?
In general, the world of numbers was simple, elegant, and everyone was happy. Suddenly, the already-mentioned theorem, bearing the name of Pythagoras, became one of the sources of the trouble that arose: one of the most important achievements that have come down to us. Unfortunately, the evidence of Pythagoras himself is unknown to us. The oldest that has come down to us is given in the "Beginnings" of Euclid and dates back to the 3rd century. BC Let me remind you that Pythagoras himself lived in the 6th century. BC
Fragment from Vatican Manuscript Number 190 dating from the 10th century. AD (entirely here ):

The proof of Euclid is far from the simplest. There is reason to believe that he knew the way and simpler, but for methodological reasons he brought precisely this option, which, in addition to the Pythagorean theorem itself, showed some other interesting ideas.
However, back to the Pythagoreans.
Just imagine the simplest thing: a square with sides of unit length. If we denote the length of its diagonal , then by the Pythagorean theorem we get:
, then by the Pythagorean theorem we get:

and, accordingly:

By itself, this is not a problem. From the point of view of the Pythagoreans, then we just needed to find integers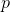 and
and 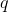 , such that
, such that
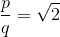
It was at this “simple” moment that everything stalled. And tightly. This stop continued until one wise guy (allegedly Gippas of Metapont, also a Pythagorean), suddenly proved that such numbers do not exist. All evil comes from very smart people, as you know. According to legend, this scientific achievement shocked colleagues so much that, in commemoration of the recognition of scientific merit, Hippasa was immediately thrown overboard the ship on which he was sailing at the time of his mathematical insight. But there was nothing to spoil respected people, to undermine the foundations of such a lovingly fostered and highly profitable philosophical system.
Nowadays, numbers that cannot be represented as the relations of two integers are called irrational.
For some time, the Pythagoreans even held the fact of irrationality in secret. However, you can’t hide the sewn in the bag, and the truth quite quickly (by historical standards) found the way out.
in secret. However, you can’t hide the sewn in the bag, and the truth quite quickly (by historical standards) found the way out.
To prove irrationality is not at all difficult.
is not at all difficult.
Let there exist such that
that
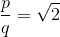
Moreover, we assume that at least one of the numbers is odd. If this is not so, the numerator and denominator of the fraction can always be reduced by 2 (the required number of times).
is odd. If this is not so, the numerator and denominator of the fraction can always be reduced by 2 (the required number of times).
Then we get:

from here:

Thus, is an even number. But then
is an even number. But then 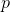 - even.
- even.
By the condition of oddness of at least one of the numbers , we get that
, we get that 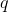 - is odd.
- is odd.
Due to parity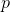 , we can write
, we can write
 ,
,
where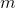 - a whole.
- a whole.
But then:

from here

But this means that - even, and therefore even and
- even, and therefore even and 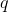 . Contradiction.
. Contradiction.
A number cannot be represented as the ratio of two integers.
cannot be represented as the ratio of two integers.
It remains to add that - this is not at all some strange freak. It can be shown that there are more irrational numbers than rational ones, fundamentally more. By the way, the more or less relationship in the world of infinite sets is itself very counter-intuitive. But that's another story.
- this is not at all some strange freak. It can be shown that there are more irrational numbers than rational ones, fundamentally more. By the way, the more or less relationship in the world of infinite sets is itself very counter-intuitive. But that's another story.
PS. I take this opportunity to congratulate the Khabrovsk citizens on coming. Good luck in the new year!
UPD In connection with the discussion that erupted in the comments, I want to note the following: to be honest, rational numbers are introduced as a field of quotientsrings of integers. Whether to use a set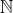 or a set as a multiplicative system
or a set as a multiplicative system 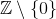 is a purely matter of taste, which does not affect the result. The question is which of the school pseudo-definitions is “true” is decided by the Ministry of Education, and this process has a very distant relation to mathematics.
is a purely matter of taste, which does not affect the result. The question is which of the school pseudo-definitions is “true” is decided by the Ministry of Education, and this process has a very distant relation to mathematics.
The troubles among the Pythagoreans did not begin immediately. The scientific school founded by Pythagoras ended up badly, but today's story is not about the vigorous pogrom that was inflicted on the Pythagoreans by the people thankful for enlightenment, but more about spiritual ups and downs.
The term "scientific school" in relation to the organization founded by Pythagoras is a kind of euphemism. Taking a healthy look at its structure and applied technologies, Pythagoreanism should be safely attributed to totalitarian cults, which was quite in the spirit of the times (however, it is always in the spirit of the times, an eternal classic). There was a separation into degrees of initiation, and a complex system of rituals with prohibitions (for example, well-known, such as “do not eat beans” or “do not bite off a whole bun”), and a complex philosophical creed. Hello to Ron Hubbard and his comrades. There is nothing new under the sun.
In general, during the life of Pythagoras, his “school” was a solid enterprise, besides having significant and ever-growing political influence.
In general, the philosophy of Pythagoras had a significant impact on Western culture (including us). Many ideas found their development in classical Greek philosophy, and already everyone knows about the Pythagorean theorem. The expression "harmony of the spheres", by the way, also dates back to the Pythagoreans.
One of the essential elements of their philosophy was the idea that any number can be represented as the ratio of two integers, that is, in the form of a simple fraction. In this they, in particular, saw the perfection of the nature of number. Moreover, it seemed quite obvious. In modern mathematics, such numbers are called rational, and their number is indicated by
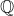 . Now pause for a few seconds, think, where does it generally follow that this is not so? Can you give an argument that would be convincing enough for the ancient Greek? Well, or at least convincing enough for yourself personally?
. Now pause for a few seconds, think, where does it generally follow that this is not so? Can you give an argument that would be convincing enough for the ancient Greek? Well, or at least convincing enough for yourself personally? In general, the world of numbers was simple, elegant, and everyone was happy. Suddenly, the already-mentioned theorem, bearing the name of Pythagoras, became one of the sources of the trouble that arose: one of the most important achievements that have come down to us. Unfortunately, the evidence of Pythagoras himself is unknown to us. The oldest that has come down to us is given in the "Beginnings" of Euclid and dates back to the 3rd century. BC Let me remind you that Pythagoras himself lived in the 6th century. BC
Fragment from Vatican Manuscript Number 190 dating from the 10th century. AD (entirely here ):

The proof of Euclid is far from the simplest. There is reason to believe that he knew the way and simpler, but for methodological reasons he brought precisely this option, which, in addition to the Pythagorean theorem itself, showed some other interesting ideas.
However, back to the Pythagoreans.
Just imagine the simplest thing: a square with sides of unit length. If we denote the length of its diagonal
 , then by the Pythagorean theorem we get:
, then by the Pythagorean theorem we get: 
and, accordingly:

By itself, this is not a problem. From the point of view of the Pythagoreans, then we just needed to find integers
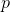 and
and 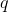 , such that
, such that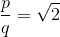
It was at this “simple” moment that everything stalled. And tightly. This stop continued until one wise guy (allegedly Gippas of Metapont, also a Pythagorean), suddenly proved that such numbers do not exist. All evil comes from very smart people, as you know. According to legend, this scientific achievement shocked colleagues so much that, in commemoration of the recognition of scientific merit, Hippasa was immediately thrown overboard the ship on which he was sailing at the time of his mathematical insight. But there was nothing to spoil respected people, to undermine the foundations of such a lovingly fostered and highly profitable philosophical system.
Nowadays, numbers that cannot be represented as the relations of two integers are called irrational.
For some time, the Pythagoreans even held the fact of irrationality
To prove irrationality
Let there exist such
 that
that 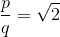
Moreover, we assume that at least one of the numbers
 is odd. If this is not so, the numerator and denominator of the fraction can always be reduced by 2 (the required number of times).
is odd. If this is not so, the numerator and denominator of the fraction can always be reduced by 2 (the required number of times). Then we get:

from here:

Thus,
 is an even number. But then
is an even number. But then 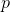 - even.
- even. By the condition of oddness of at least one of the numbers
 , we get that
, we get that 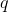 - is odd.
- is odd. Due to parity
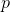 , we can write
, we can write  ,
, where
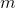 - a whole.
- a whole. But then:

from here

But this means that
 - even, and therefore even and
- even, and therefore even and 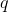 . Contradiction.
. Contradiction. A number
It remains to add that
PS. I take this opportunity to congratulate the Khabrovsk citizens on coming. Good luck in the new year!
UPD In connection with the discussion that erupted in the comments, I want to note the following: to be honest, rational numbers are introduced as a field of quotientsrings of integers. Whether to use a set
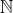 or a set as a multiplicative system
or a set as a multiplicative system 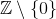 is a purely matter of taste, which does not affect the result. The question is which of the school pseudo-definitions is “true” is decided by the Ministry of Education, and this process has a very distant relation to mathematics.
is a purely matter of taste, which does not affect the result. The question is which of the school pseudo-definitions is “true” is decided by the Ministry of Education, and this process has a very distant relation to mathematics.