Model of the connection of atoms into molecules
The previous publication received a negative assessment with the wording “why post such elementary things here”. Therefore, I immediately warn that this material is intended primarily for schoolchildren who begin to study chemistry. And also for those who did not understand this subject in their school years. I would post an article on a popular specialized resource for schoolchildren if it existed.
And yes, I am aware of the existence of an atomic model in which the electron is a probability wave located near the nucleus. But, as a rule, it is difficult for a student to imagine how probability can combine atoms into molecules. Therefore, I state "on the fingers."
The connection of atoms into molecules
Our world does not exist in the form of separate atoms, they somehow connect with each other. Which one?
Take two hydrogen atoms. Each of them contains one proton and one electron, so the total charge of each of these atoms is zero.
The Coulomb law
F = k * q1 * q2 / r ^ 2
tells us that neutral bodies should not be attracted to each other
(q1 = 0, q2 = 0) .
So, hydrogen (and any other chemical element) should exist only in the form of atoms, and never combine into molecules. In fact, hydrogen atoms always combine in pairs. Why?
Let's take two pieces of metal, and arrange them in parallel at a small distance from each other.

Both segments contain the same number of protons and electrons, therefore, the total charge of each of them is equal to zero. So, they have no reason for mutual attraction.
We know that in metals a part of the external electrons leaves their atoms and freely walks between the ions (abandoned atoms) of the crystal lattice of the metal. And these electrons are distributed, on average, evenly.
Imagine that we somehow managed to move part of these free electrons to the left side of the lower segment of the metal. Moreover, in its right side there will be a deficit of electrons.

We got the so-called dipole: the left side of the segment is negatively charged, the right is positive. Excellent. And what will happen in the upper segment? We know that the same charges repel each other, while the opposite ones attract. Consequently, the electrons of the upper segment, starting from the electric fields of the electrons of the lower segment, will go to the right side. That is, the picture of the distribution of electrons in these two segments of metals will become a mirror:
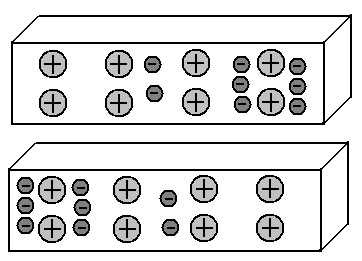
This effect of charged objects on neighboring objects, leading to a redistribution of charges in them, is called electrostatic induction.
Now the most interesting thing: the positively charged atomic nuclei in the left side of the upper segment were opposite electrons collected in the left side of the lower segment. And the opposite charges are attracted. So, the left parts of the segments will begin to attract each other!
The same thing will happen on the right side of the segments - only mirrored. And the right ends of the segments will also be attracted to each other. Wonderful, isn't it? The redistribution of charges inside one of the segments of the conductors has led to the mutual attraction of these two segments!
But what happens if we now move the free electrons of the lower segment to its right end? Then the free electrons of the upper segment will move to the left end. That is, moving electrons back and forth in one of the segments, we make the electrons of the neighboring segment move, which is not connected in any way with the first! Such an effect of the movement of electrons in one conductor on the movement of electrons in an adjacent conductor is called electrodynamic induction.
Although this does not apply to our topic, we note that we have studied in a somewhat simplified form how the antenna and receiver work during radio transmission.
We can arrange these two pieces of metal differently - with the ends facing each other:

If we manage to move the electrons, for example, to the right side of the left segment, the electrons of the right segment, starting from them, will also move to the right side of the right segment:

And in this case, these two segments of metal will begin to attract each other, since their near ends have opposite charge. It should be especially noted that in the second variant of the arrangement of the segments, the force of their mutual attraction will be weaker, since only their opposite ends are attracted, while in the first variant of the arrangement of the segments, both left and right are attracted to each other the ends.
But how does this relate to the bonding of atoms? Let's look at the hydrogen atom. It has an electron moving around the nucleus. And if the second hydrogen atom is nearby, this electron will cause the neighbor’s electron to move in the same way as they moved in our metal segments - while the electron of one of the atoms is on one side of the nucleus of its atom, the neighboring one will be forced to be on the opposite side of its atom.
Here, of course, the influence is not one-sided, but mutual - both the first electron affects the second, and the second affects the first. But the most important thing is that these two atoms will be attracted in the same way as two pieces of metal were attracted in the second variant of their mutual arrangement (with ends facing each other).
The essence is the same: the electrons are kept away from each other, allowing opposite charges to be attracted to each other. Imagine that the electron of one of the atoms was between the nuclei of two neighboring atoms, while the electron of the neighboring atom was at the opposite, remote point of the orbit:

Now we have a negatively charged electron located between two positively charged atomic nuclei. The nuclei of both atoms are attracted to this electron. Thus, an electron currently binds two atoms.
The distance between the nuclei of atoms is greater than the distance from each of the nuclei to the electron located between them. And we remember that the force of interaction of charges is inversely proportional to the square of the distance between them. Therefore, at the moment, the force of attraction of the nuclei to the electron is greater than the mutual repulsion of the nuclei.
But the electrons are constantly moving, and therefore after a while the first electron leaves the space between the nuclei, but the second electron moves there. At this moment, the role of the binder passes to the electron of the second atom (moment 3 in the figure below).

Note that at the time points shown in Figures 2 and 4, there are no electrons between the nuclei of atoms. At these moments, the nuclei repel each other. For this reason, the distance between atoms varies - it constantly changes during the rotation of electrons around the nuclei, but its average length, called the bond length, is preserved. The bond length - the distance between the nuclei of atoms - is individual for each pair of types of atoms combined into a molecule.
The electrons of these two atoms in the formed hydrogen molecule try to be as far apart as possible, just as they did in metal segments. Due to this, their synchronization occurs - their location relative to each other at each revolution around the nuclei is approximately the same.
This is somewhat reminiscent of the collective performance of a waltz, when the pairs rotate at the same speed so that neither the ladies nor the gentlemen will ever be next to each other, but always alternate:

This article is an excerpt from the book “Understanding Chemistry” .
Holy Uncertainty and Holy Probability The
quantum theory states that it is impossible to simultaneously determine the exact place of an electron in space and its momentum (direction and speed of its motion). Therefore, it is believed that around the nucleus of an atom there are certain places (areas) in which the probability of detecting an electron is high. These areas are called electron orbitals.
This theory is not difficult to explain on a household example. Suppose you live in an apartment in which there is a bedroom, a kitchen and a bathroom. If you spend 90% of the time in the bedroom, 8% of the time in the kitchen, and 2% of the time in the bathroom, then your orbital can be considered the bedroom and the kitchen, since the probability of finding you in the bathroom is very low. After 100 observations of you at different points in time, the observer is likely to find you in 90 cases in the bedroom, and in 8 cases in the kitchen. And by these figures it will come to a conclusion about the area of your habitat.
Now about why it is impossible to simultaneously determine the place of an electron in space and its speed, and the direction of motion. It’s even easier. The fact is that speed can only be measured on a certain intervaldistance traveled. Dividing the length of this segment by the time for which it has passed, we can find out the speed of movement. But we cannot consider a segment of space as the location of the body. Location is the exact coordinate of the body.
Imagine a fly flying in a dark room. By illuminating the room with a very short flash of light, we can see the place where the fly is currently located. But in order to understand where and at what speed it flies, we will have to turn on the light for a longer time. Then we will see a change in the position of the fly over time and will be able to estimate the speed of this change. But in this case, we can no longer indicate the exact location, in which the fly was during the measurement of its speed, since during this time it moved a certain distance. That is the whole point of the uncertainty principle.
Electrons moving around atomic nuclei very quickly change the speed and direction of motion, so it is impossible to say exactly where they are at a given time and where they are moving.
And in the model discussed above, electrons move like arrows in a clock. And this cannot but cause the righteous anger of the adherents of the Holy Uncertainty and the Holy Probability.
However, the fact that we cannot say exactly where this or that electron is located, and to which of the atoms it “belongs” does not at all change the electrostatic mechanism of the binding of atoms. It is impossible to bind two protons otherwise than by placing an electron between them. No probability or uncertainty can connect atoms into a molecule. And it perfectly demonstrates the molecular hydrogen ion H2 +. This ion has neither an electron doublet, nor compensation of the spins of paired electrons, nor overlapping of electron clouds, however, this ion exists and is stable.
In addition, do not forget that this is just a model, and its “explanatory” capabilities are limited, as are the capabilities of any other models. For example, it (it seems) does not explain why hydrogen atoms cannot join into long chains like H3, H4, etc.
However, we can assume that due to the fact that the electronic orbitals in the hydrogen molecules are displaced to the center of the molecule, they do not "protrude" from its ends, and therefore neighboring hydrogen molecules are not able to cling to each other using the electron synchronization mechanism.
And yes, I am aware of the existence of an atomic model in which the electron is a probability wave located near the nucleus. But, as a rule, it is difficult for a student to imagine how probability can combine atoms into molecules. Therefore, I state "on the fingers."
The connection of atoms into molecules
Our world does not exist in the form of separate atoms, they somehow connect with each other. Which one?
Take two hydrogen atoms. Each of them contains one proton and one electron, so the total charge of each of these atoms is zero.
The Coulomb law
F = k * q1 * q2 / r ^ 2
tells us that neutral bodies should not be attracted to each other
(q1 = 0, q2 = 0) .
So, hydrogen (and any other chemical element) should exist only in the form of atoms, and never combine into molecules. In fact, hydrogen atoms always combine in pairs. Why?
Let's take two pieces of metal, and arrange them in parallel at a small distance from each other.

Both segments contain the same number of protons and electrons, therefore, the total charge of each of them is equal to zero. So, they have no reason for mutual attraction.
We know that in metals a part of the external electrons leaves their atoms and freely walks between the ions (abandoned atoms) of the crystal lattice of the metal. And these electrons are distributed, on average, evenly.
Imagine that we somehow managed to move part of these free electrons to the left side of the lower segment of the metal. Moreover, in its right side there will be a deficit of electrons.

We got the so-called dipole: the left side of the segment is negatively charged, the right is positive. Excellent. And what will happen in the upper segment? We know that the same charges repel each other, while the opposite ones attract. Consequently, the electrons of the upper segment, starting from the electric fields of the electrons of the lower segment, will go to the right side. That is, the picture of the distribution of electrons in these two segments of metals will become a mirror:

This effect of charged objects on neighboring objects, leading to a redistribution of charges in them, is called electrostatic induction.
Now the most interesting thing: the positively charged atomic nuclei in the left side of the upper segment were opposite electrons collected in the left side of the lower segment. And the opposite charges are attracted. So, the left parts of the segments will begin to attract each other!
The same thing will happen on the right side of the segments - only mirrored. And the right ends of the segments will also be attracted to each other. Wonderful, isn't it? The redistribution of charges inside one of the segments of the conductors has led to the mutual attraction of these two segments!
But what happens if we now move the free electrons of the lower segment to its right end? Then the free electrons of the upper segment will move to the left end. That is, moving electrons back and forth in one of the segments, we make the electrons of the neighboring segment move, which is not connected in any way with the first! Such an effect of the movement of electrons in one conductor on the movement of electrons in an adjacent conductor is called electrodynamic induction.
Although this does not apply to our topic, we note that we have studied in a somewhat simplified form how the antenna and receiver work during radio transmission.
We can arrange these two pieces of metal differently - with the ends facing each other:

If we manage to move the electrons, for example, to the right side of the left segment, the electrons of the right segment, starting from them, will also move to the right side of the right segment:

And in this case, these two segments of metal will begin to attract each other, since their near ends have opposite charge. It should be especially noted that in the second variant of the arrangement of the segments, the force of their mutual attraction will be weaker, since only their opposite ends are attracted, while in the first variant of the arrangement of the segments, both left and right are attracted to each other the ends.
But how does this relate to the bonding of atoms? Let's look at the hydrogen atom. It has an electron moving around the nucleus. And if the second hydrogen atom is nearby, this electron will cause the neighbor’s electron to move in the same way as they moved in our metal segments - while the electron of one of the atoms is on one side of the nucleus of its atom, the neighboring one will be forced to be on the opposite side of its atom.
Here, of course, the influence is not one-sided, but mutual - both the first electron affects the second, and the second affects the first. But the most important thing is that these two atoms will be attracted in the same way as two pieces of metal were attracted in the second variant of their mutual arrangement (with ends facing each other).
The essence is the same: the electrons are kept away from each other, allowing opposite charges to be attracted to each other. Imagine that the electron of one of the atoms was between the nuclei of two neighboring atoms, while the electron of the neighboring atom was at the opposite, remote point of the orbit:

Now we have a negatively charged electron located between two positively charged atomic nuclei. The nuclei of both atoms are attracted to this electron. Thus, an electron currently binds two atoms.
The distance between the nuclei of atoms is greater than the distance from each of the nuclei to the electron located between them. And we remember that the force of interaction of charges is inversely proportional to the square of the distance between them. Therefore, at the moment, the force of attraction of the nuclei to the electron is greater than the mutual repulsion of the nuclei.
But the electrons are constantly moving, and therefore after a while the first electron leaves the space between the nuclei, but the second electron moves there. At this moment, the role of the binder passes to the electron of the second atom (moment 3 in the figure below).

Note that at the time points shown in Figures 2 and 4, there are no electrons between the nuclei of atoms. At these moments, the nuclei repel each other. For this reason, the distance between atoms varies - it constantly changes during the rotation of electrons around the nuclei, but its average length, called the bond length, is preserved. The bond length - the distance between the nuclei of atoms - is individual for each pair of types of atoms combined into a molecule.
The electrons of these two atoms in the formed hydrogen molecule try to be as far apart as possible, just as they did in metal segments. Due to this, their synchronization occurs - their location relative to each other at each revolution around the nuclei is approximately the same.
This is somewhat reminiscent of the collective performance of a waltz, when the pairs rotate at the same speed so that neither the ladies nor the gentlemen will ever be next to each other, but always alternate:

This article is an excerpt from the book “Understanding Chemistry” .
Holy Uncertainty and Holy Probability The
quantum theory states that it is impossible to simultaneously determine the exact place of an electron in space and its momentum (direction and speed of its motion). Therefore, it is believed that around the nucleus of an atom there are certain places (areas) in which the probability of detecting an electron is high. These areas are called electron orbitals.
This theory is not difficult to explain on a household example. Suppose you live in an apartment in which there is a bedroom, a kitchen and a bathroom. If you spend 90% of the time in the bedroom, 8% of the time in the kitchen, and 2% of the time in the bathroom, then your orbital can be considered the bedroom and the kitchen, since the probability of finding you in the bathroom is very low. After 100 observations of you at different points in time, the observer is likely to find you in 90 cases in the bedroom, and in 8 cases in the kitchen. And by these figures it will come to a conclusion about the area of your habitat.
Now about why it is impossible to simultaneously determine the place of an electron in space and its speed, and the direction of motion. It’s even easier. The fact is that speed can only be measured on a certain intervaldistance traveled. Dividing the length of this segment by the time for which it has passed, we can find out the speed of movement. But we cannot consider a segment of space as the location of the body. Location is the exact coordinate of the body.
Imagine a fly flying in a dark room. By illuminating the room with a very short flash of light, we can see the place where the fly is currently located. But in order to understand where and at what speed it flies, we will have to turn on the light for a longer time. Then we will see a change in the position of the fly over time and will be able to estimate the speed of this change. But in this case, we can no longer indicate the exact location, in which the fly was during the measurement of its speed, since during this time it moved a certain distance. That is the whole point of the uncertainty principle.
Electrons moving around atomic nuclei very quickly change the speed and direction of motion, so it is impossible to say exactly where they are at a given time and where they are moving.
And in the model discussed above, electrons move like arrows in a clock. And this cannot but cause the righteous anger of the adherents of the Holy Uncertainty and the Holy Probability.
However, the fact that we cannot say exactly where this or that electron is located, and to which of the atoms it “belongs” does not at all change the electrostatic mechanism of the binding of atoms. It is impossible to bind two protons otherwise than by placing an electron between them. No probability or uncertainty can connect atoms into a molecule. And it perfectly demonstrates the molecular hydrogen ion H2 +. This ion has neither an electron doublet, nor compensation of the spins of paired electrons, nor overlapping of electron clouds, however, this ion exists and is stable.
In addition, do not forget that this is just a model, and its “explanatory” capabilities are limited, as are the capabilities of any other models. For example, it (it seems) does not explain why hydrogen atoms cannot join into long chains like H3, H4, etc.
However, we can assume that due to the fact that the electronic orbitals in the hydrogen molecules are displaced to the center of the molecule, they do not "protrude" from its ends, and therefore neighboring hydrogen molecules are not able to cling to each other using the electron synchronization mechanism.
