Thermoacoustic Basics

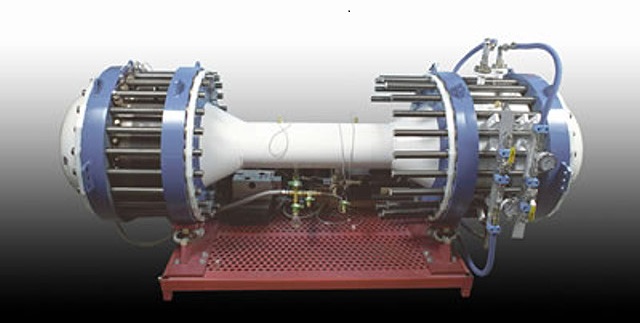
Fig. 1. Thermo-acoustic refrigerator THEAC-25 with a traveling wave (left) and thermo-acoustic refrigerator with a standing wave Triton C-10c (right)

Previous parts “1 article” , “2 article” .


1) Thermoacoustic engine, refrigerator and heat pump

1.1) Engine
In a thermoacoustic engine, thermal energy is converted into acoustic energy. Such a process is called a direct thermoacoustic effect.



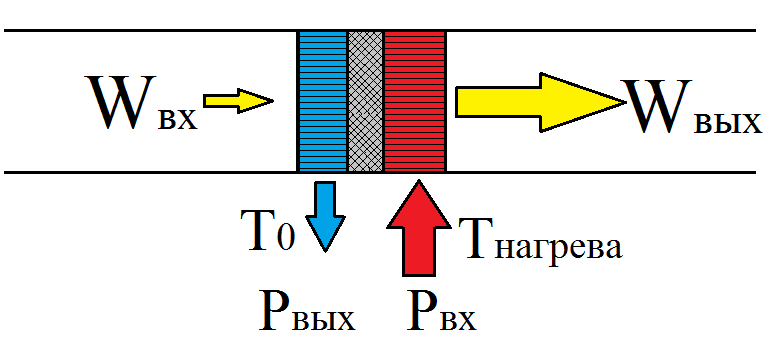
Fig. 2. Amplification of the acoustic wave power in the engine

heat exchanger. A wave of small power Win arrives at the input of the heat exchanger and amplifies, passing through the engine heat exchanger to the value of Wout. In order to amplify the wave, it is necessary to expend thermal energy. The thermal energy Pin is supplied at the heating temperature Tnag. Not all thermal energy can be converted into acoustic energy, since the maximum conversion efficiency of any heat engine is limited by the efficiency of the Carnot cycle. Therefore, it is necessary to dump part of the thermal power Pout into the environment. The temperature at which heat is removed is equal to - To. Please note that in the engine, the direction of temperature increase in the heat exchanger and the direction in which the acoustic power grows are the same.

1.2) Refrigerator

In the refrigerator, the reverse thermoacoustic effect is realized. That is, the opposite is happening, compared with the engine. A powerful acoustic wave Win arrives at the input of the heat exchanger apparatus of the refrigerator, which attenuates in the heat exchanger to the value of Wout. The loss of wave power is to create a temperature difference between the heat exchangers.



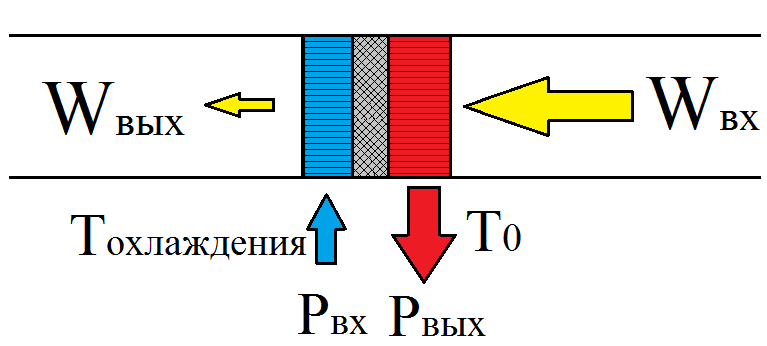
Fig. 3. Creating a temperature difference during wave attenuation

One of the heat exchangers starts to heat up, and the other starts to cool. That is, an acoustic wave takes away heat from one heat exchanger and transfers it to another. In this case, it is necessary to discharge the thermal power Pout from the heating heat exchanger into the environment, and the thermal power Pin, which is the useful thermal power of the refrigerator, enters the cold heat exchanger. The refrigerator cools an object to the temperature Tohl.

1.3) Heat pump A

heat pump is essentially the same as a refrigerator, with the only difference being that heat taken from the environment is considered useful in the refrigerator, and power delivered to the environment in the heat pump (for various needs).



Fig. 4. Also the creation of a temperature difference during wave attenuation, only heating power, not cooling, is considered to be useful power.

Note that both in the refrigerator and in the heat pump, the direction in which the temperature in the heat exchanger decreases is opposite to the direction in which the acoustic wave power decreases , which indicates that the inverse thermoacoustic effect is realized. The acoustic wave entering the input can be created using the engine, or using a powerful speaker or piston connected to a linear electric motor.

2) Devices with a standing wave and devices with a traveling wave

According to the type of wave in the resonator, thermoacoustic devices are divided into two types, devices with a traveling wave and devices with a standing wave.
Let's see what are the differences between a traveling and a standing acoustic wave.

2.1) Running wave



Gifka 1. Graph of gas pressure, velocity and image of its displacement in a traveling wave. A

traveling wave runs along the resonator on the gif 1 to the right. In a traveling wave, pressure and gas velocity fluctuations are in phase. In this case, energy is transferred in the direction of wave motion. The pink ovals in gif 1 depict diagrams of the dependence of pressure on the coordinate of elementary gas portions at various points inside the resonator. The acoustic power carried by the wave to the right is numerically equal to the area of ​​the pink oval, that is, the area of ​​the PX diagram. As you can see, the ovals at different points are the same, which indicates that the wave power does not change when moving along the resonator. That is, the attenuation of the wave when moving along the resonator is not taken into account here.

A traveling wave can propagate in a resonator, which is a looped tube. In this case, the wavelength corresponding to the resonant frequency of such a resonator will be equal to the length of the pipe itself.



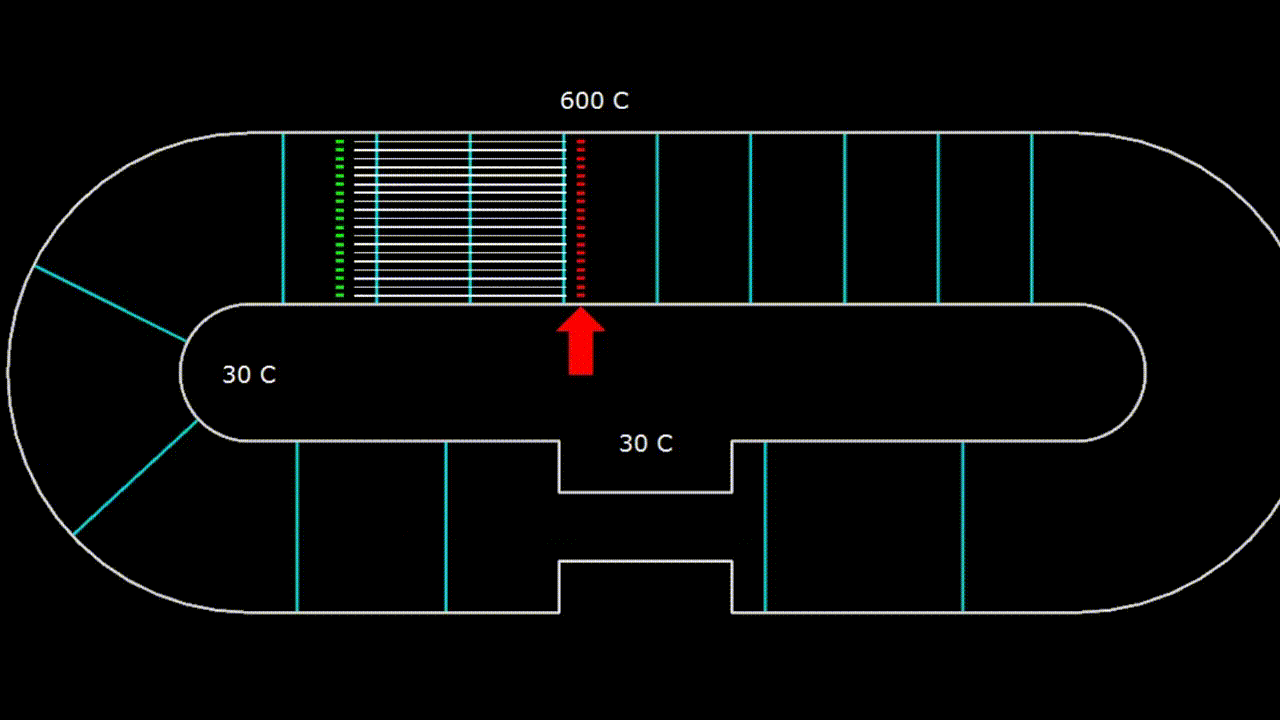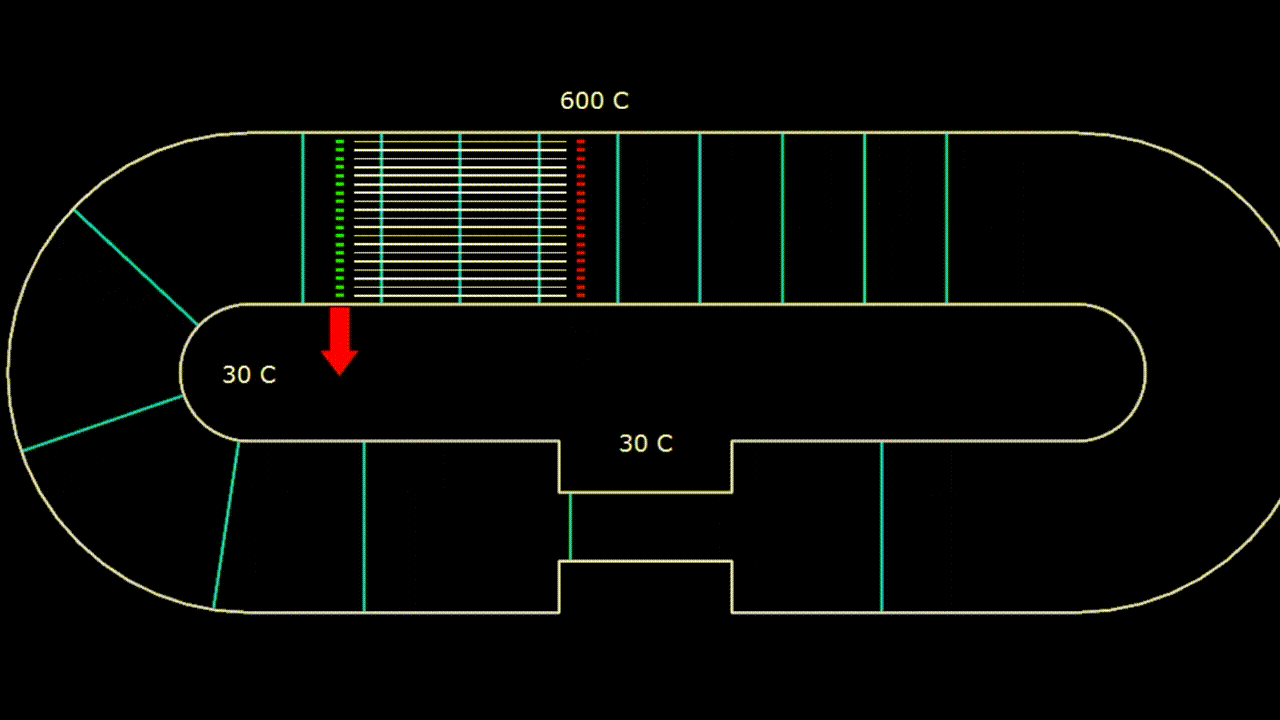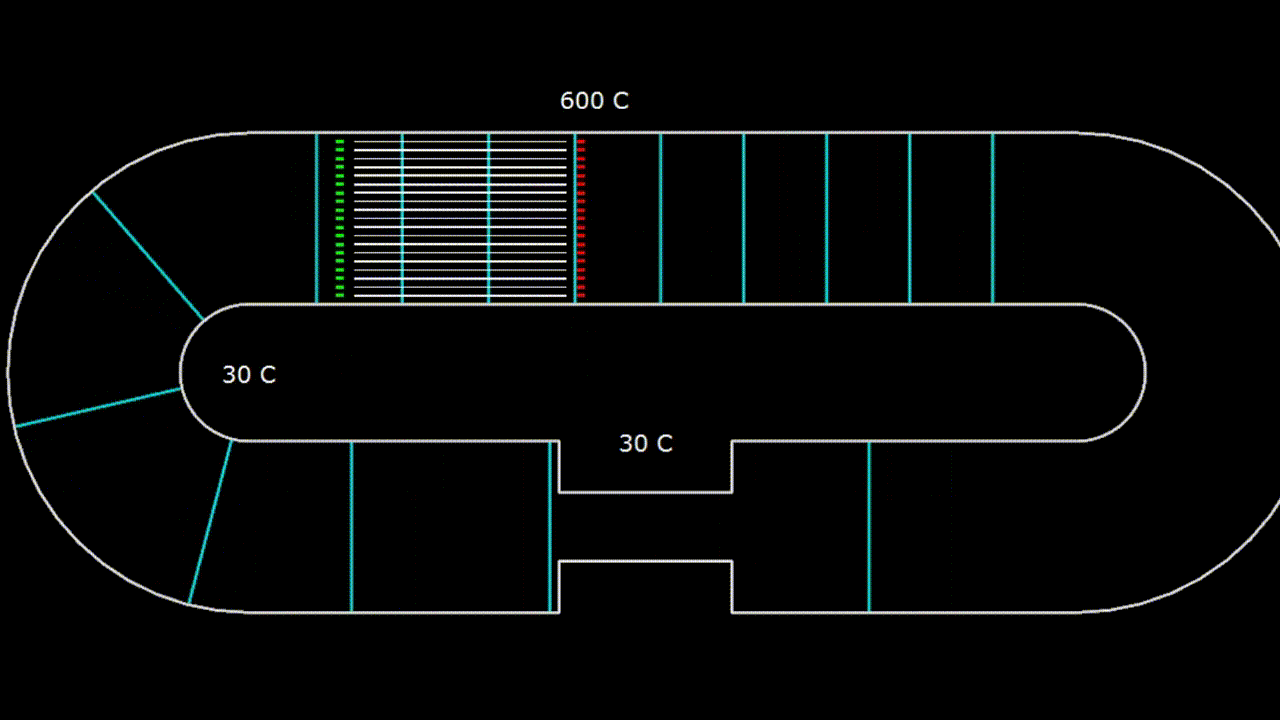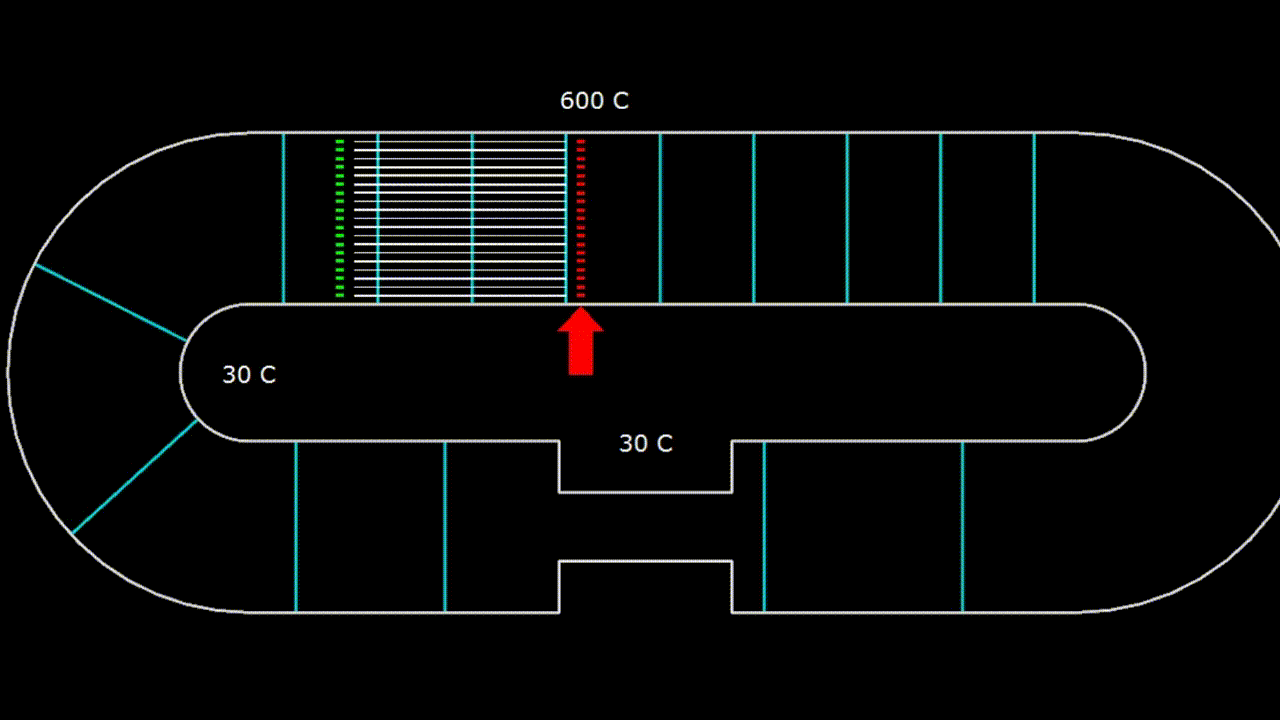
GIF 2. A device with a traveling wave

2.2) Standing wave

A standing wave is the sum of two traveling waves propagating in opposite directions. Such a wave can occur during reflection, from any object and return to the sound source.



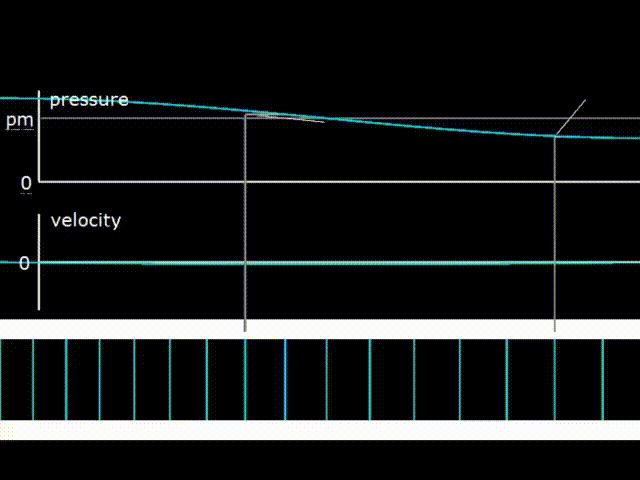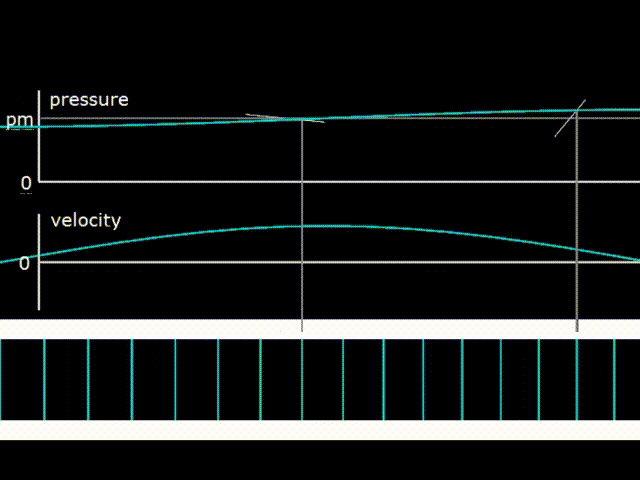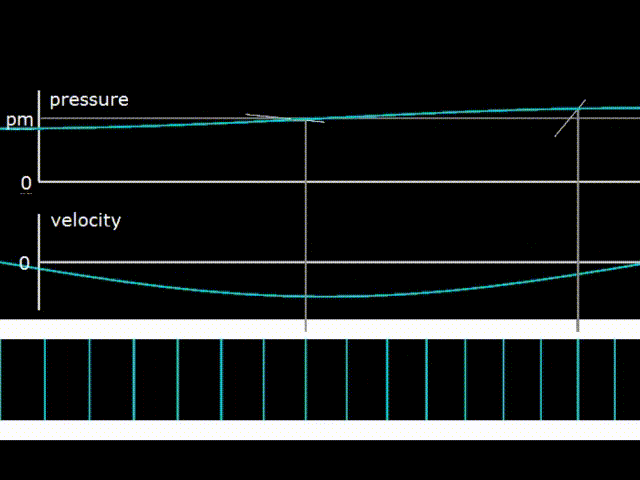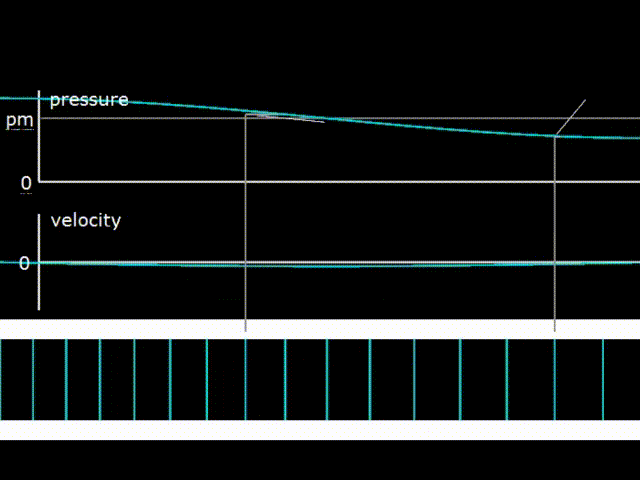
GIF 3. Graph of gas pressure, velocity and image of its displacement in a standing wave.

On GIF 3 a standing wave is shown in a half-wave resonator, that is, in a resonator with a length equal to half the wavelength. It can be imagined that the resonator below on GIF 3 is a pipe plugged with plugs on both sides. In this case, someone, for example, shakes the pipe, and the gas inside hangs between the two ends of the pipe. Since the ends of the pipe are plugged, the gas velocity on the surface of the plugs can only be zero (as can be seen on the velocity graph). That is, speed nodes appear at the ends of the pipe. At the same time, it is clear that the largest pressure fluctuations in amplitude (anti-nodes or pressure antinodes) will be observed on the plugs, and the pressure unit (the point where there are no vibrations) will be in the middle of the pipe.

In a standing wave, the phase difference between pressure fluctuations and velocity fluctuations is 90 degrees. In this case, the PX diagrams at all points of the resonator are lines, that is, figures that have no area. Accordingly, energy transfer in a standing wave does not occur, either to the right or to the left. But the wave itself naturally has energy.

A standing wave in a half-wave resonator can be created by placing a speaker or piston at one of its ends, producing oscillations at the resonant frequency of the resonator. And by placing an additional heat exchanger in the resonator, you can create a thermoacoustic refrigerator.



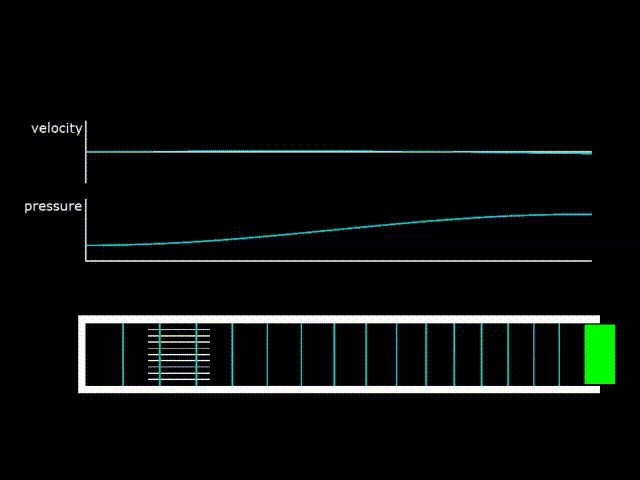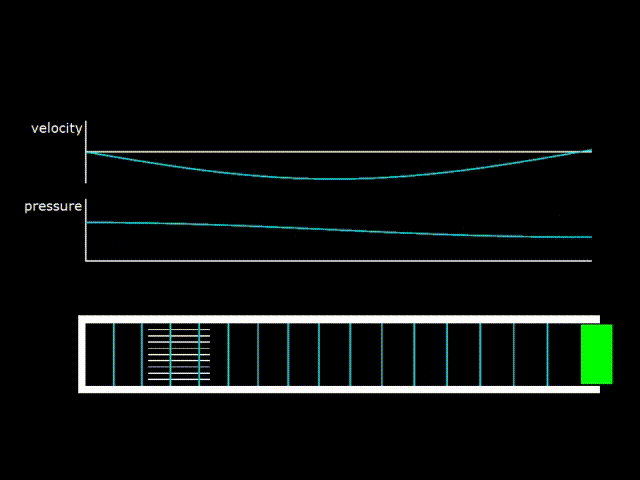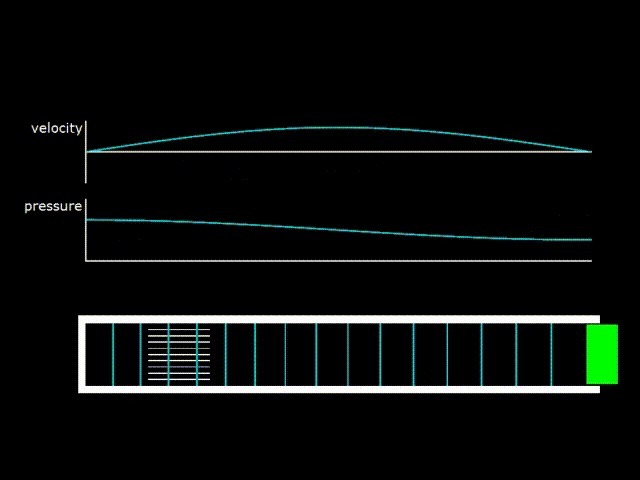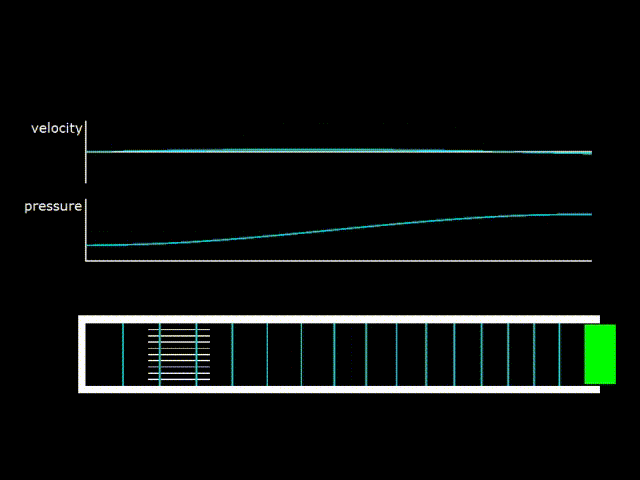
GIF 4. A standing wave in a half-wave resonator. On the left side of the resonator is the so-called stack - an analog of the regenerator in a traveling wave engine.

Due to the dissipation of acoustic energy in the resonator and in the heat exchanger, the resulting wave will not be purely standing. Constant energization from the piston will be needed. On GIF 4, it can be seen that, since the piston oscillates, the gas at the piston oscillates with it. There is a transfer of acoustic energy from the piston to the resonator, which compensates for the energy loss in the resonator. Thus, even though the resulting wave is very close to a standing wave, it is a sum of a standing and traveling wave when examined more finely.

In real thermoacoustic devices, there is also never a purely traveling or purely standing wave. A wave is always something intermediate, but if the wave in the device is very similar to a standing wave, then the device is called a device with a standing wave, and if the wave is similar to a traveling wave, then it is called a device with a traveling wave.

3) Main dimensions

3.1) Case
length The length of the case - resonator of a thermoacoustic device is determined by the wavelength. It is even better to say the opposite, that the length of the resonator housing determines the wavelength in the resonator.

In standing wave devices, the case length is usually half the wavelength. For example, for a typical frequency of 300 Hz for a typical type of device, the length of the case when working in air will be about 0.56 meters, and when working with helium 1.65 meters.



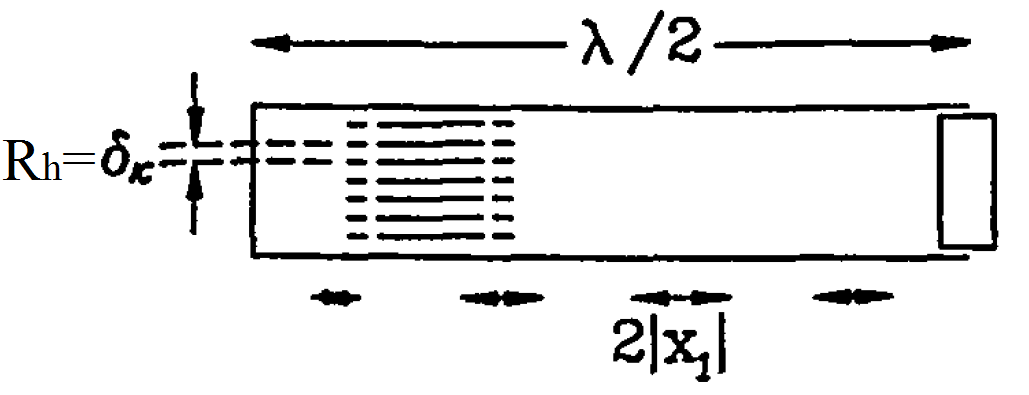
Fig. 5. The main dimensions of a device with a standing wave

In devices with a traveling wave, the wavelength is approximately equal to the length of the body. The typical oscillation frequency in such devices is 100 Hz, while the length of the case when working in air will be 3.4 meters, and when working on helium - 10 meters.



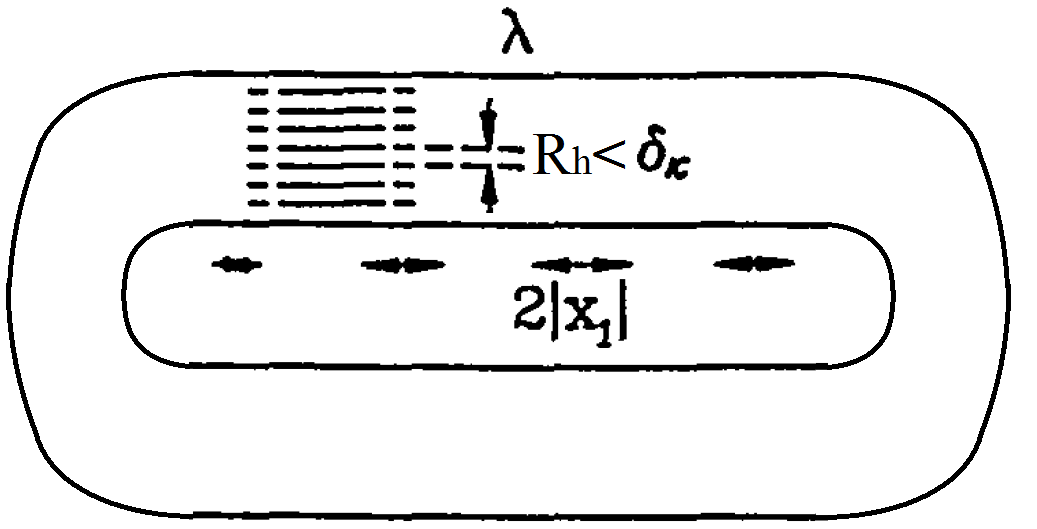
Fig. 6. The main dimensions of the traveling wave device

3.2) Case
diameter The case diameter is determined based on the required device power. Power increases with increasing diameter of the device in proportion to the cross-sectional area of ​​the housing, since in proportion to the cross-sectional area, the power of the heat exchanger increases.

The resonator is a conventional pipe, preferably with smooth walls.



Gifka 5. Interaction of an oscillating gas with the resonator wall

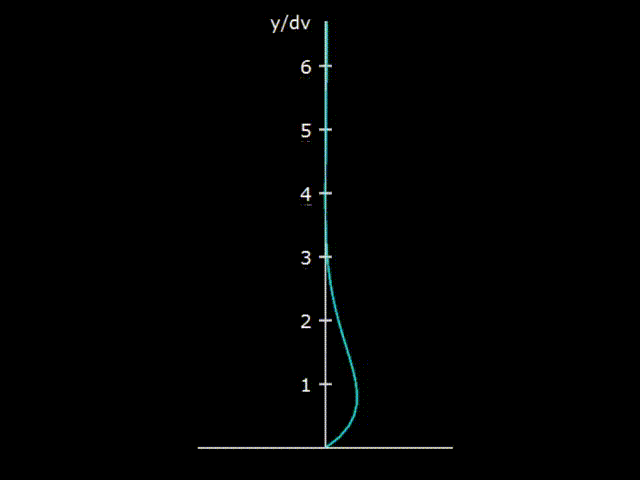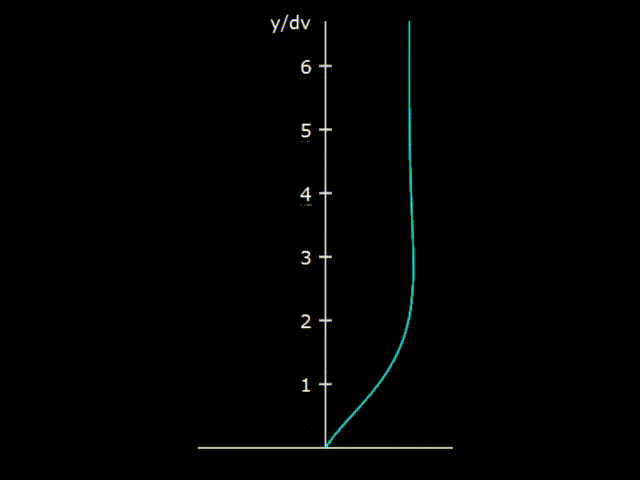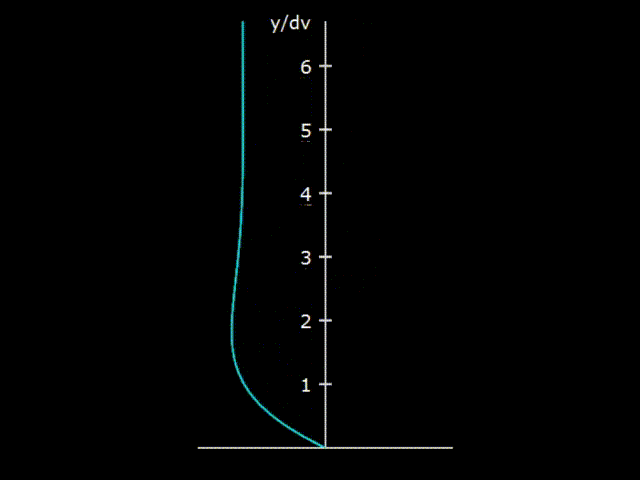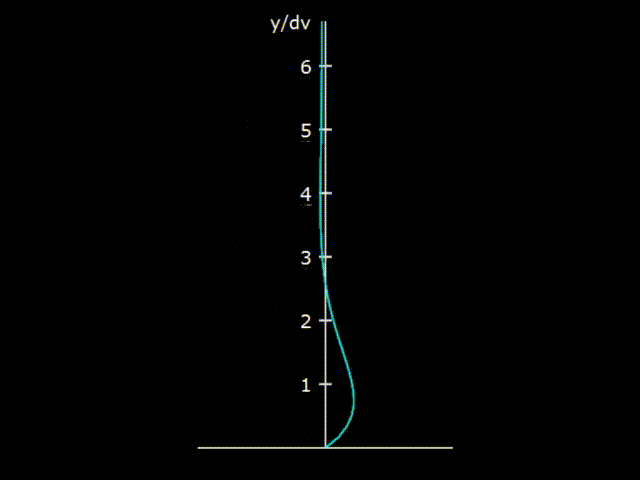
If we consider the propagation of an acoustic wave in a resonator of a sufficiently large diameter (about a centimeter or more), it turns out that the gas in the wave interacts with the resonator wall not in its entire volume, but only in a small boundary a layer located at the cavity wall. On gifka 5, it is shown that during gas oscillations an unusual deformation of the gas velocity due to friction against the wall is formed near the cavity wall. On the wall surface, the gas velocity is zero, which is usually accepted as a boundary condition in most hydrodynamic problems.

Depth of viscous penetration The
vertical axis on the graph is marked in the so-called values ​​of viscous penetration δν.

The depth of viscous penetration is an estimate of the size of the layer actively interacting with the wall of the body. For example, for an acoustic wave propagating in air with normal conditions at a frequency of 70 Hz, the depth of viscous penetration is 0.27 mm. On GIF 5, it can be seen that the interaction of the wall and gas is observed at values ​​greater than the depth of viscous penetration, but, nevertheless, the region of sufficiently active interaction of the wave with the wall has a value of only about 1 mm. In the center of the resonator, ordinary acoustic vibrations are observed, exactly the same as if there were no resonator at all. Accordingly, the scattering of acoustic energy due to friction against the walls occurs only in a narrow boundary layer near the wall.

Thermal penetration depth
In the acoustic wave, gas compresses and expands, while gas temperature fluctuates due to alternating adiabatic heating and cooling. This happens in a wave propagating in free space. When the wave moves in the cavity, the wave interacts with the cavity wall, and the wall temperature begins to affect the gas temperature fluctuations in the acoustic wave.

In the same way as for the viscous interaction with the wall, there is also a quantity for thermal interaction that characterizes the size of the gas layer actively thermally interacting with the wall. This quantity is called - thermal penetration depth δκ. Fluctuations in the gas temperature near the wall are deformed in the same way as the gas velocity in the previous example. So if you just say that now on GIF 5 fluctuations occur not in gas velocity but in temperature and that now the vertical axis is marked not in the depths of viscous penetration, but in thermal depths, then GIF 5 will be true for temperature fluctuations. Numerically, the depth of thermal penetration is always greater than the depth of viscous.

What conclusions can be drawn from all this?

Well, firstly, with a sufficiently large diameter of the resonator, the wave almost does not viscously or thermally interact with the resonator. The resonator only sets the direction of the wave and the type of wave. It follows that in order to transfer and take away heat energy from the gas, the size of the channels (pores, openings, slots) in the heat exchanger must be somewhere in the region of the thermal penetration, but in no case is much larger than this value.

Then, since the depths of viscous and thermal penetration are almost equal for any gases and for any frequencies, thermoacoustic devices are doomed to have losses associated with friction of the gas on the surface of the heat exchanger.

3.3) Channel dimensions in a heat exchanger
For traveling wave devices, in order to achieve maximum efficiency, the hydraulic radius of the pores in the heat exchanger must be less than the thermal penetration depth Rh <δk in order to ensure good thermal contact between the gas and the surface of the heat exchanger. This condition follows from the equations of thermoacoustics. For the regenerator, this condition is especially important. Usually the optimal value of the hydraulic radius of the pores of the regenerator, somewhere from 3.5 to 6 times less than the depth of thermal penetration. The size of the pores in the heat exchangers affects the device much less than the size of the pores in the regenerator, therefore, it is usually preferred to increase the size of the pores (channels) in the heat exchangers, relative to the pores in the regenerator, for ease of manufacture.

The thermoacoustic equations on the other hand tell us that in devices with a standing wave, the value of the hydraulic radius of the pores of the stack (analogue of the regenerator in devices with a traveling wave) should be approximately equal to the depth of thermal penetration in the gas. That is, in a device with a standing wave, the pore size in the stack should be somewhere in 3.5-6 times greater than in a device with a traveling wave, ceteris paribus. The size of the pores in the heat exchangers of devices with a standing wave does not affect the efficiency of the device as much as the size of the pores in the stack, as well as in devices with a traveling wave.

3.4) Length of heat exchangers and regenerator
In an acoustic wave, each elementary portion of gas performs harmonic oscillations relative to its equilibrium position with amplitude X1 (see Fig. 5 and Fig. 6). The value of the optimal length of the regenerator or stack is usually greater than the gas displacement 2 | X1 | (greater than the doubled amplitude of the deviation of the elementary portion of the gas from the equilibrium position). If the typical value for the offset is 1 cm, then the regenerator or stack may have a length of 1 cm to 5 cm, depending on the operating temperature. The length of the heat exchangers is in the same order as for the regenerator.

4) Thermodynamic cycle in devices with a standing wave and in devices with a traveling

4.1) Engine and refrigerator with a standing wave
The thermodynamic cycle implemented in the stack of a device with a standing wave is closest to the Brighton cycle, which is implemented in a gas turbine engine.

Engine



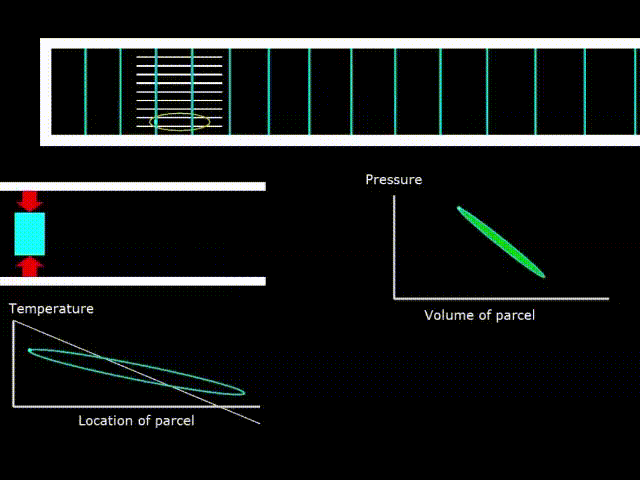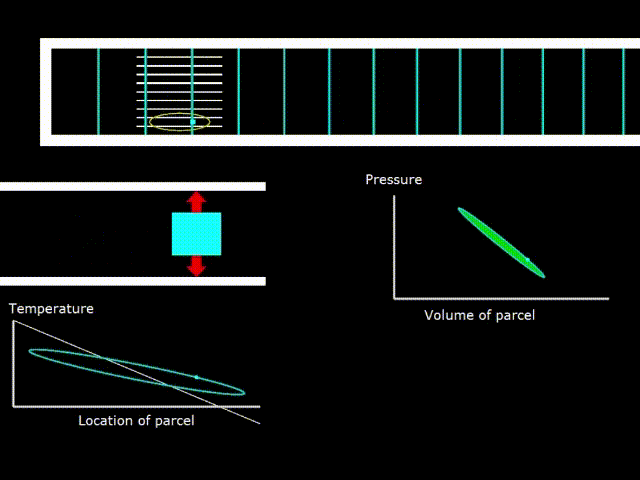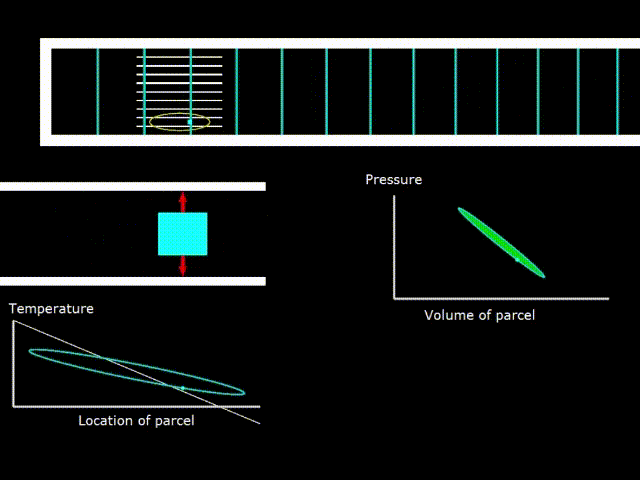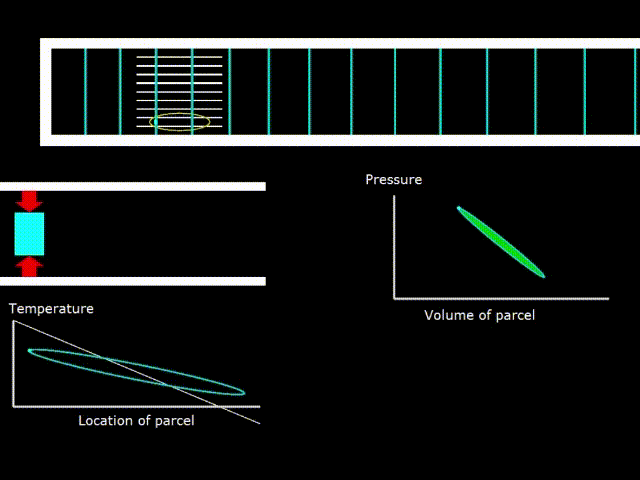
GIF 6. Thermodynamic cycle in an engine with a standing wave.

On GIF 6. oscillations of the elementary volume of gas between stack plates are shown. Gas, by compressing and expanding, changes its temperature (graph in the lower left corner). The graph of the temperature versus coordinate is a figure similar to an oval (green line). The white line on the graph indicates the surface temperature of the stack. You can see that there is a temperature gradient along the length of the stack. That is, the temperature decreases linearly when moving from the left to the right end of the stack.

If the white line of the stack temperature has a slope on the graph greater than the slope of the oval - gas temperature graph, then the device works like an engine.

The PV diagram is shown in the middle right - the dependence of pressure on volume in an elementary portion of gas. The oval area in the diagram is numerically equal to the work done on gas in the case of an engine and the work done on gas in the case of a refrigerator (heat pump).

Since when working with a standing wave, the optimal size of the stack channels is approximately equal to the depth of thermal penetration, the thermal contact of the gas and the solid surface is not ideal and the temperature of the gas and stack, at any particular point in the stack, can differ from each other. If the thermal contact between the gas and the stack were ideal, then the graphs of the temperature of the gas and the stack coincided, since the gas would instantly take the temperature of the surface of the stack, at whatever point it appears.

Critical temperature gradient in the stack



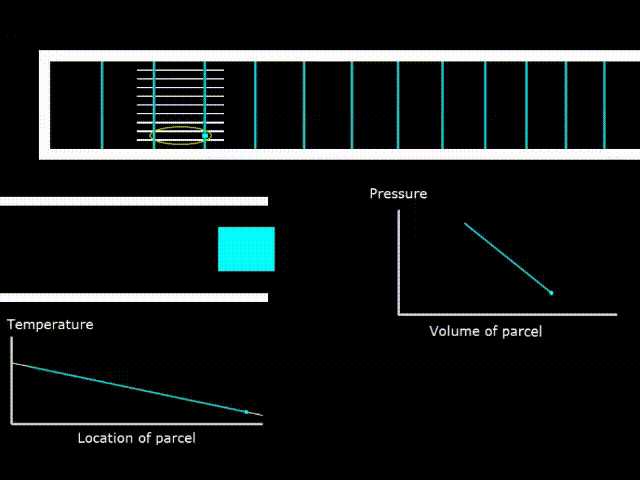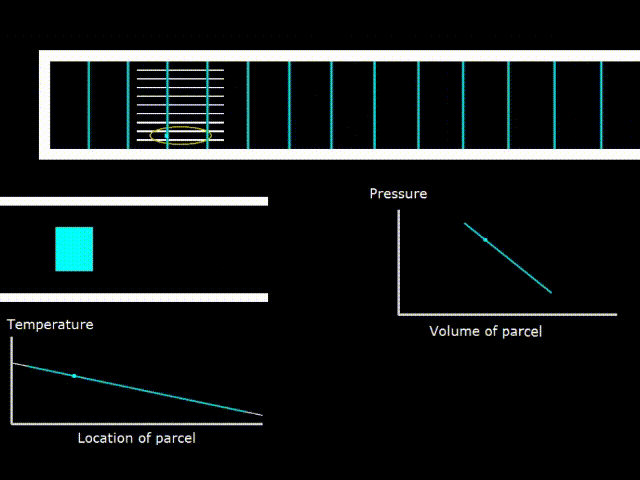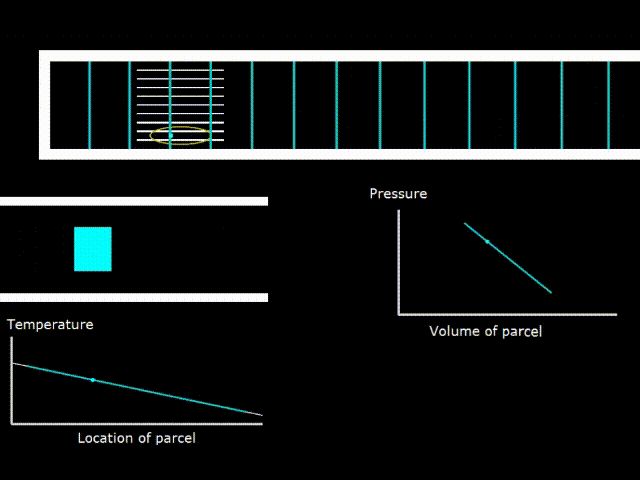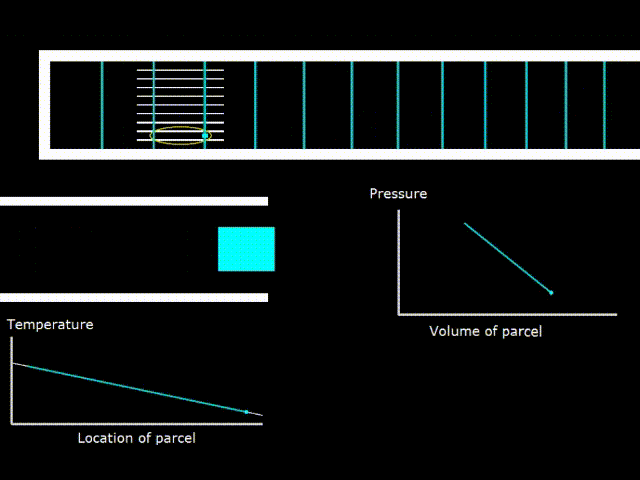
Gifka 7. Critical temperature gradient in the stack

Now we take the engine and begin to reduce the temperature difference on the stack, while in any way preserving the amplitude of the acoustic wave, for example, using a speaker. At the same time, it’s too soon or late, a state occurs in which the temperature in the elementary portion of the gas in the wave begins to fluctuate so that its temperature begins to coincide with the temperature of the surface of the stack, wherever this portion of gas is located (GIF 7. green and white lines in the temperature graph match).

In this case, no work is done on the stack (the PV diagram is a line - a figure that has no area)

The temperature gradient in the stack at which the case described above is realized is called the critical temperature gradient for this particular wave. A device with a critical temperature gradient is absolutely useless for practical use. It occupies a position exactly between the engine and the refrigerator. Nevertheless, it is convenient to compare devices with respect to it in order to find out whether it is an engine or a refrigerator.

Refrigerator



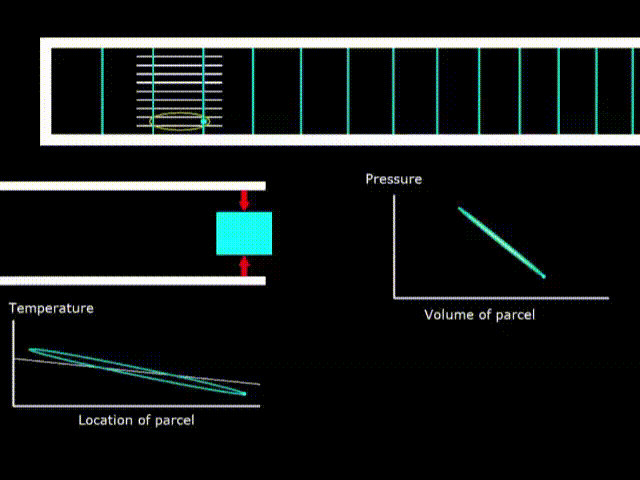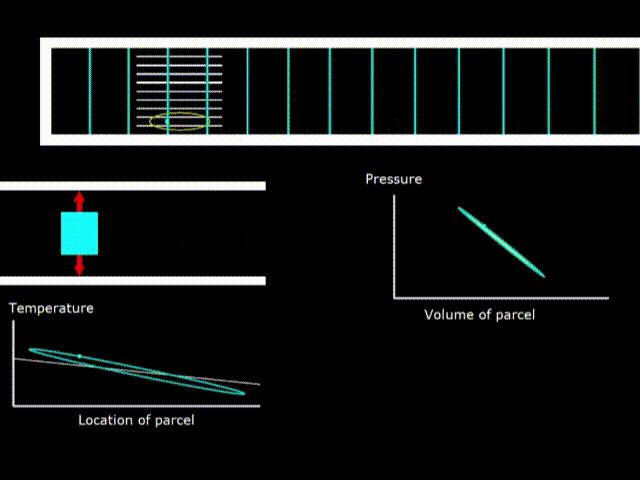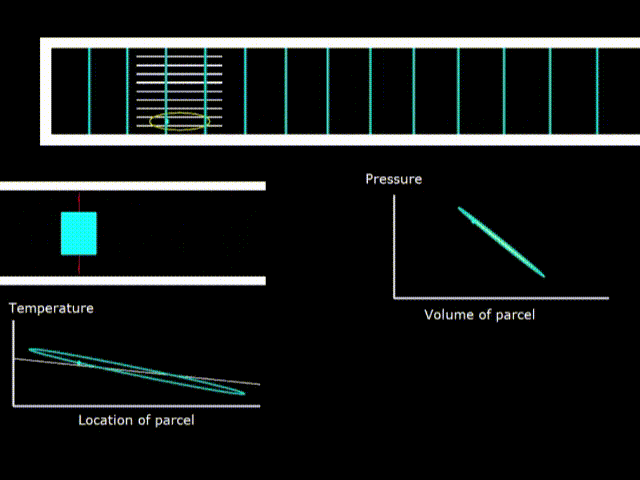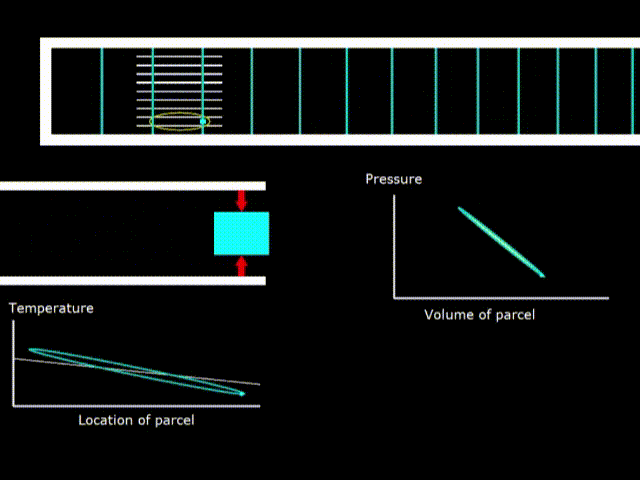
Gifka 8. Thermodynamic cycle in a refrigerator with a standing wave.

If the stack temperature slope is less than that of the oval gas temperature, then the device works like a refrigerator.

Please note that the rotation of the green dot in the diagrams of the engine and the refrigerator goes in opposite directions, which means that in one case work is done on gas, and in the other, gas does work.

What needs to be done to turn the refrigerator into an engine? You must either increase the temperature gradient in the stack while maintaining the amplitude of the acoustic wave, or reduce the amplitude of the wave while maintaining the temperature gradient.

4.2) Running wave engine and refrigerator
The thermodynamic cycle implemented in the regenerator of the traveling wave device is closest to the Stirling cycle, which is implemented in the engine of the same name.

In devices with a traveling wave, the case of ideal thermal contact between the gas and the surface of the regenerator is realized, due to the small optimal pore size.



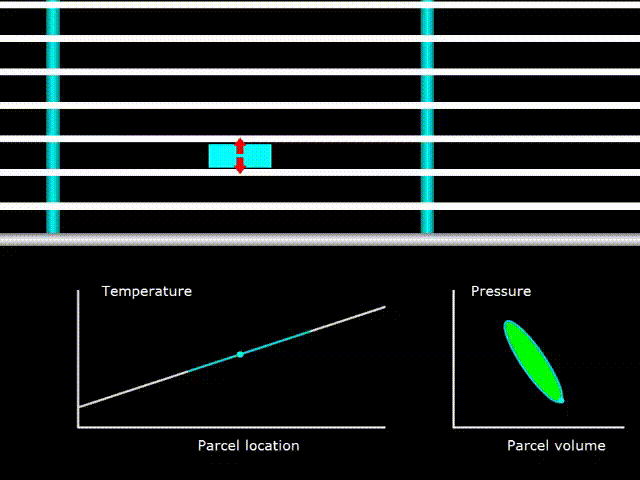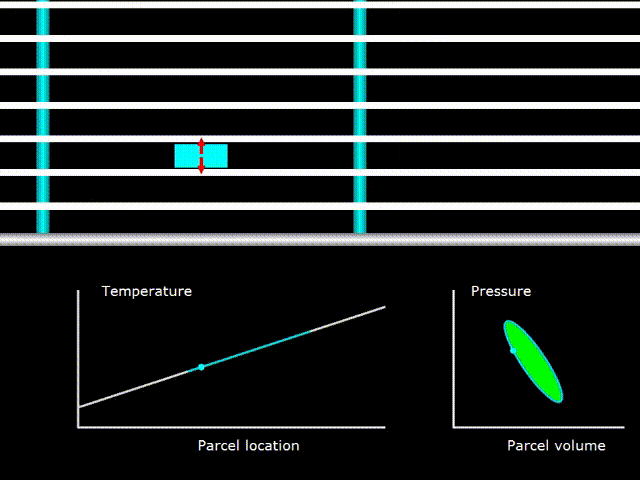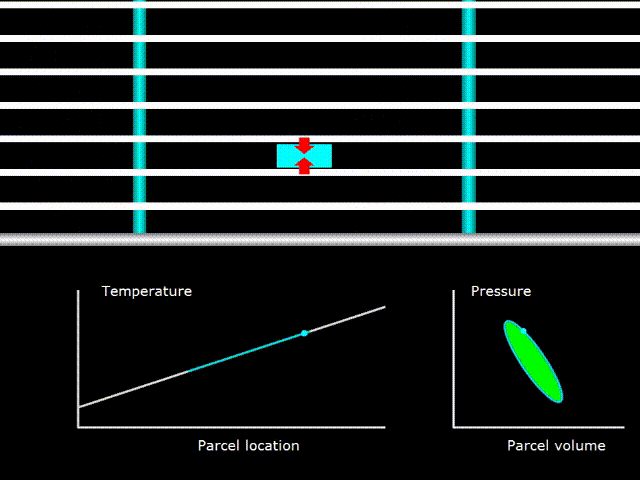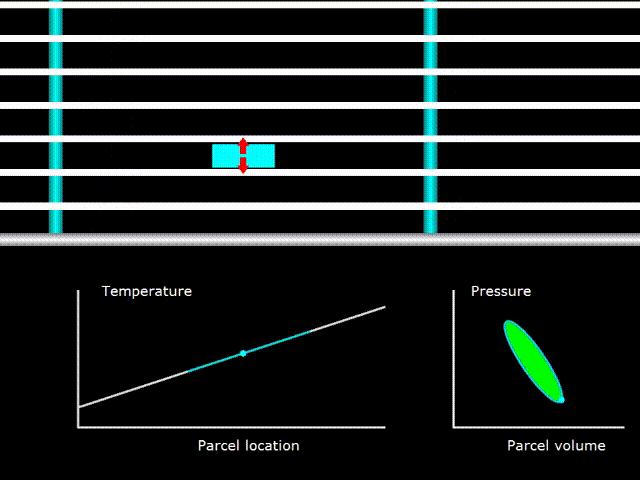
Gifka 9. Thermodynamic cycle in a traveling wave engine

Here the gas temperature (green line on the temperature graph) coincides with the temperature of the regenerator at all its points (white line on the temperature graph). The PV diagram in the lower right corner indicates that gas is being worked on.

It should be understood that although the temperature graphs of the gas and the regenerator are the same, but this is not a device with a critical temperature gradient in the sense described earlier. In devices with a standing wave, it was necessary to select the necessary temperature gradient for a given wave so that it coincided with temperature fluctuations in the acoustic wave. In traveling wave devices, because the pores in the regenerator are very small, good thermal contact between the regenerator and the gas is always ensured. Therefore, a critical temperature gradient in traveling wave devices always exists and this term loses any meaning here. How then is work done on gas? Indeed, with a critical temperature gradient, in the case of a device with a standing wave, there was no work. The thing is

For a refrigerator with a traveling wave, the graphs will look exactly the same as on GIF 9, except that the green dot on the PV diagram will rotate in the opposite direction, which will indicate that the gas is doing work, and not working on gas .

In conclusion, for everyone who wants to learn more about thermoacoustics, I want to recommend the book by G. Swift, who made a huge contribution to thermoacoustics by working at the Los Alamos National Laboratory:

Swift GW Thermoacoustic engines and refrigerators: a short course. Los Alamos: Los Alamos National Laboratory, 1999. 179 p. URL: download link

I also attach animations of thermoacoustic processes created by G. Swift's team:
download link. To view animations, you need to unzip the archive, put it in the program files folder on your hard drive (otherwise for some reason they do not work). All animations for windows are in the EXEs folder.

In this article, I retold only a small part of what is in this book, without using mathematics. In the original, everything is much more interesting.