Summing up the online contest for the game Blotto
A few days ago I had an online contest for the game .
1,199 people took part in the online contest, and there were more than 5,000 decisions, among which 61 were attempts to get around the system by finding vulnerabilities. This is very cool, thanks to everyone who participated.
This game is a type of “ Colonel Blotto's games ”. Two players take part in it. Each has the same number of resources that must be placed on the NxM field. The winner is the one who takes the most cells (i.e. the number of your resources on it is greater than that of the opponent). This is the condition for our task
When I started this experiment, I still did not know that this game does not have an ideal solution, but thanks to the comments I could better understand this topic.
Let's start with the winners of this game.

The best decision scored 4121 victories - a very good result. But what would happen if only TOP-100 solutions participated in the game?
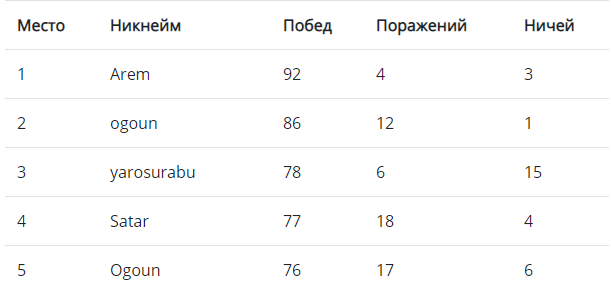
Surprisingly, it did not even enter the top5 (at 8th place), and the first place was taken by the decision from 68th place.
This just shows that there is no better solution. The rest of the results can be viewed at game.pavlukhinlab.com
But what else can you learn?
The players put the largest numbers in the center, on average, all the players filled the first line with large numbers, while the best results put more emphasis on the bottom line.


average values for all games (left) and TOP-100 (right)
Well, and more examples of the best strategies:
The best strategies selected 5 main cells, the remaining cells were filled with small values. Which is logical, because to win it is enough to take 5 cells.
Well, now a few graphs.
Probability distribution of numbers:
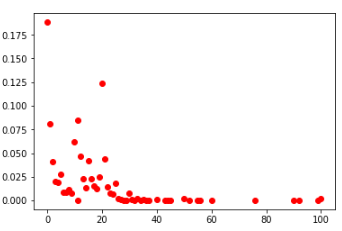
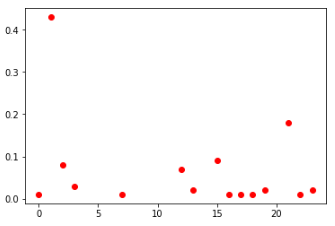
second cells among all players (left) and TOP-100 (right)
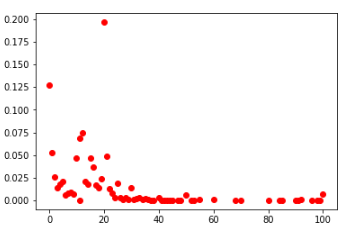
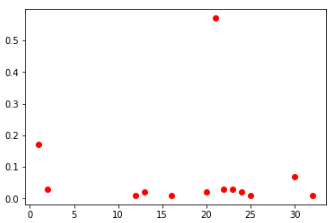
central cells among all and TOP-100
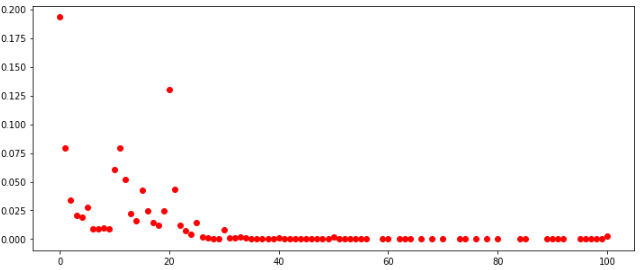
distribution of numbers for all games
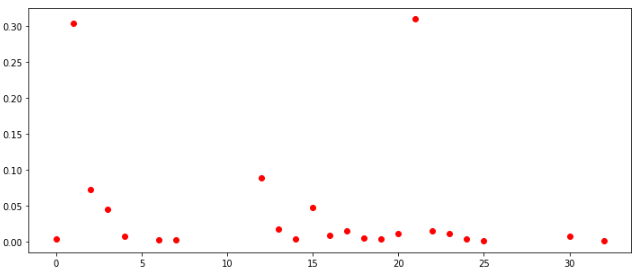
distribution of numbers in TOP-100
If you look at the distribution of all cells among all players, then they look they are generally similar. It can also be concluded that in these conditions it makes no sense to fill the cells with values greater than 30.
This is where the study comes to an end - that’s all I can extract from this data. Perhaps one of you will be able to offer something else that you can check - I'm waiting for you in the comments. Look for impersonal games here .
Py.Sy. In a previous post, I talked about a neuron who was learning to play this game. Unfortunately, none of this has yet been achieved. Two neurons decided that the best strategy is to throw zeros at the exit and enjoy the draw. But maybe I'll figure it out again and make a separate post on the mistakes that I made when creating a neuron, and, possibly, success.
1,199 people took part in the online contest, and there were more than 5,000 decisions, among which 61 were attempts to get around the system by finding vulnerabilities. This is very cool, thanks to everyone who participated.
This game is a type of “ Colonel Blotto's games ”. Two players take part in it. Each has the same number of resources that must be placed on the NxM field. The winner is the one who takes the most cells (i.e. the number of your resources on it is greater than that of the opponent). This is the condition for our task
Condition
1. Two are playing.
2. The game field is a 3 by 3 board.
3. Each player has an army of 100 space marines.
4. Before the battle at night, each side secretly places its troops in a random way on 9 cells. On each cell, you can put any integer number of space marines from 0 to 100.
5. In the morning the battle for the next planet begins. On each of 9 cells, the player who has more Astartes on this cell wins. For a victory on each of 9 cells 1 point is given. If the same number is on a certain square, the battle on this square ends in a draw, and both players get 0.5 points.
6. The battle is won by the one who won more fields. If both players have won 4.5 fields, the battle ends in a draw.
2. The game field is a 3 by 3 board.
3. Each player has an army of 100 space marines.
4. Before the battle at night, each side secretly places its troops in a random way on 9 cells. On each cell, you can put any integer number of space marines from 0 to 100.
5. In the morning the battle for the next planet begins. On each of 9 cells, the player who has more Astartes on this cell wins. For a victory on each of 9 cells 1 point is given. If the same number is on a certain square, the battle on this square ends in a draw, and both players get 0.5 points.
6. The battle is won by the one who won more fields. If both players have won 4.5 fields, the battle ends in a draw.
When I started this experiment, I still did not know that this game does not have an ideal solution, but thanks to the comments I could better understand this topic.
Let's start with the winners of this game.

Decision Noone 4173

The best decision scored 4121 victories - a very good result. But what would happen if only TOP-100 solutions participated in the game?

Surprisingly, it did not even enter the top5 (at 8th place), and the first place was taken by the decision from 68th place.
Arem Solution

This just shows that there is no better solution. The rest of the results can be viewed at game.pavlukhinlab.com
But what else can you learn?
The players put the largest numbers in the center, on average, all the players filled the first line with large numbers, while the best results put more emphasis on the bottom line.


average values for all games (left) and TOP-100 (right)
Well, and more examples of the best strategies:
Examples of Ogoun strategies (6 solutions in the TOP-100)



Examples of Guten strategies (8 solutions in the TOP-100)



The best strategies selected 5 main cells, the remaining cells were filled with small values. Which is logical, because to win it is enough to take 5 cells.
Well, now a few graphs.
Probability distribution of numbers:


second cells among all players (left) and TOP-100 (right)


central cells among all and TOP-100

distribution of numbers for all games

distribution of numbers in TOP-100
If you look at the distribution of all cells among all players, then they look they are generally similar. It can also be concluded that in these conditions it makes no sense to fill the cells with values greater than 30.
This is where the study comes to an end - that’s all I can extract from this data. Perhaps one of you will be able to offer something else that you can check - I'm waiting for you in the comments. Look for impersonal games here .
Py.Sy. In a previous post, I talked about a neuron who was learning to play this game. Unfortunately, none of this has yet been achieved. Two neurons decided that the best strategy is to throw zeros at the exit and enjoy the draw. But maybe I'll figure it out again and make a separate post on the mistakes that I made when creating a neuron, and, possibly, success.
