RE: Is time travel possible?
Yesterday on Habré there appeared a post, amazing in its ignorance, " Are time travel possible ". In response to my remark about being unfamiliar with basic scientific ideas about space and time, the author printed me like this:
I have not seen such a level of militant ignorance for a long time, and it’s doubly strange to see him on Habré.
Since I, unlike the author, is a scientist , I will try to tell the curious reader what we really know about the nature of time, causality and time travel.
In classical mechanics, time is considered absolute and unchanged in the sense that during the transition from one reference system to another, time intervals do not change:
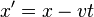



Here (x, y, z, t) are the coordinates in the old system, (x ', y', z ', t') - coordinates in the new system. Hereinafter, it is assumed (to simplify the formulas) that one system moves relative to another parallel to the x axis with speed v.
This is the so-called Galilean transformations - what happens to the coordinates when changing the frame of reference. In the Galilean sense, the “stream of time" is one throughout the Universe, and the time coordinates of all objects are the same. At the same time, classical mechanics does not in any way interpret the uniqueness of the arrow of time; Moreover, the very concept of the movement of time is not included in Newtonian formulas in any way.
In classical mechanics, we ourselves introduce a movement from the past to the future. Suppose we have a set of material points (coordinates and speeds) and acting forces. Next, we ask ourselves some dt interval and see how the system will evolve over time. No one is stopping us from moving in the opposite direction and keeping track of what happened to the system in the past.
However, "time travel" - i.e. the movement of one particular object on the t scale to the past is forbidden by Newtonian mechanics (see above - there is one time flow for the entire Universe).
The situation became much more fun when Maxwell formulated his electrodynamics, and then Einstein, in an attempt to solve the contradictions between electrodynamics and classical mechanics, created the theory of relativity.
Within the framework of the special theory of relativity in the transition from one inertial reference system to another, we use already just such transformations:
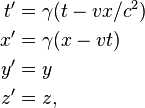 ,
,
where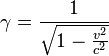 .
.
Here, as is easy to see, each reference system has its own “time scale”. Within the framework of SRT, neither the spatial nor the time interval between points is preserved - only the difference of their squares is preserved:
ds 2 = c 2 dt 2 - dx 2 - dy 2 - dz 2 .
What is written here? This description of a special four-dimensional pseudo-Euclidean space - the so-called Minkowski space - in which the distance between the points is equal to the differencesquares of coordinate differences (and not the sum, as in ordinary Euclidean space).
From the point of view of SRT, the motion of each body is a trajectory in the four-dimensional Minkowski space - the so-called "World line".
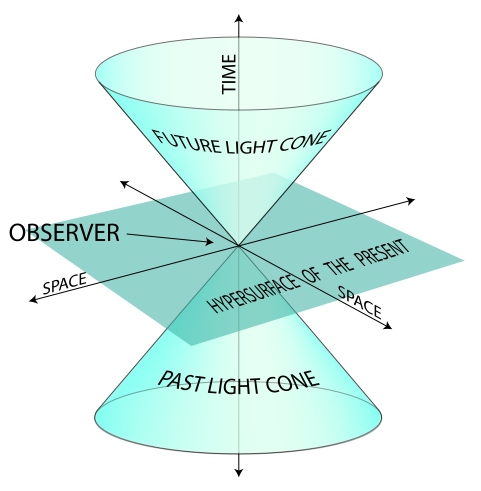
The cones in the figure are the so-called “Light cones” are the trajectories of objects moving at the speed of light.
Since now the time of each object has its own - and, moreover, it is free to change as you like, we, theoretically, can allow for “reversals” of the world line, time travel.
(Traveling in time forward to the service station is very easy - just moving at a speed close to the light - and this effect, albeit on a microscopic scale, has been successfully confirmed. Therefore, we will hereinafter talk about time travel in the sense of time travelback )
However, there are two big problems.
The first is that a material object will never be able to reach the border of the light cone - this requires an infinite amount of energy to be applied.
The second is the curious features of the Lorentzian transformations. Suppose we were able to move an object from point A to point B faster than the speed of light. Then there is surely a reference system in which the object first appears at point B and then leaves from point A. Simply put, movement faster than light is a time movement in the SRT, but only in a very non-trivial sense.
Indeed, how do you understand this “there is such a system”? It turns out that for some observers there is a movement into the past, but for some it is not. It depends solely on the speed of movement of the observer.
And, secondly, it turns out complete madness with cause and effect. Obviously, the appearance of an object at point B is a consequence of its departure from point A. But for some observers, the effect occurs later than the cause. And for some - before. We will come back to this issue.
As a result, in the service station, movement faster than light is prohibited. No, more precisely, not so: the transfer of information between objects that are outside the light cone of each other is prohibited . Otherwise, the service station is faced with insoluble contradictions.
Initially, movement faster than light is allowed - provided that systems moving faster than light do not exchange information. For example, the inflation theory suggests that at the very beginning of the big bang, the family of newborn Universes was moving away from each other at a speed many times higher than light. And there is no contradiction here - because Universes move away from each other faster than light, light from one of them will never reach the other, and information will never be transmitted.
Let us return, however, to our sheep, i.e. to a discussion about the nature of time. To admit, STO has contributed quite a bit of understanding to this issue. What is the “internal time” of an object and how is it measured? Why is the time coordinate of Minkowski space so opposed to spatial? Finally, what kind of beast is such an “inertial reference frame” and where can it be taken in reality?
Einstein also understood all these difficulties, and therefore ten years later he created the general theory of relativity (GR), which completely confused everything.
So, for starters, all the coordinates in GR (including the time) are just the numbers of points on the axis. They have no more meaning, ask any.
Further, in these coordinates for each point in space-time it is necessary to determine the so-called fundamental metric tensor g ab . What kind of beast is this? This is a 4x4 matrix that determines the distance between adjacent points. Suppose you are at some point (x 0 , x 1 , x 2 , x 3 ) and are moving in the direction (dx 0 , dx 1 , dx 2 , dx 3 ). Then you will walk the distance:
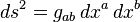
Hereinafter, summation is implied over the indices repeating from above and below, i.e. in fact, the sum of 16 components is written here.
For an ordinary Euclidean space with an orthonormal basis, the matrix g is equal to the identity. In Minkowski space, g 00 = 1, g 11 = g 22 = g 33 = -1.
In the space of general relativity, the components of the metric tensor can be absolutely anything. Those. the distance between adjacent points is an (almost) arbitrary function of the coordinate difference. Well, the coordinates are completely arbitrary, remember?
If suddenly we want to move from one coordinate system to another, all tensors (including the fundamental metric) will undergo these changes:
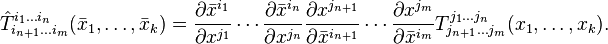
All in all, it was the easy part :). In short, in GTR you can ask for absolutely any coordinates and absolutely any reference point, the only thing you have to calculate is the fundamental metric tensor. The question about “how to measure the unique time of an object” does not seem to be worth it - number the moments of time as you like.
So, about the space-time geometry, it seems, as it turned out, we now turn to the objects in this space. From the distribution of momenta and body energies, we need to form the so-called energy-momentum tensor T ab . It is exactly the same at each point in space-time and sets the "density" of masses and energies.
Finally, we need derivatives of the fundamental tensor in all directions and derivatives (velocities) of all objects.
Now we can formulate how the geometry of space and the motion of bodies mutually influence each other.
The geometry of space changes as follows:
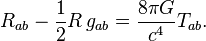
This equation is known as the Hilbert-Einstein equation. Here R ab - the so-called Ricci tensor expressed in terms of the second partial derivatives of the fundamental metric tensor. (By the way, Gregory Perelman casually proved Poincare’s theorem in his works devoted to the flows of the Ricci tensor.)
What is written here? Knowing the energy-momentum tensor, the metric tensor and the derivatives of the metric tensor, we can calculate the second derivatives of the metric tensor. Thus, we determine how energy and momentum affect the geometry of space.
Now back. To determine how the geometry of space affects the movement of objects in it, we direct for each material point the distance ds 2 → min passed by it . By revealing this condition (for example, through the Lagrange principle), we obtain expressions for the second derivatives (“accelerations”) of objects. In particular, for massless particles (light), movement occurs along a geodesic line expressed by the following equation:

Here Г a bc is the so-called. Christoffel symbols expressed through the metric tensor.
There is a rather elegant analogy that describes the meaning of such a movement in understandable terms. Imagine that we pulled a sheet and put heavy balls at some points. In these places, the sheet is bent and curved - just like space is curved in the presence of energy and momentum.
Now let's roll a light ball on our sheet. On flat areas, he will move in a straight line; but, approaching the massive points, he will bend his trajectory as if heavy balls "pulled" him. This is the effect of attraction, actually caused by the curvature of the “space” in which the ball moves.
Uhhhh Ok, to summarize how objects move in terms of GR:
- we must know the current geometry of space (metric tensor) and the position of the energies and momenta in it (energy-momentum tensor);
- in addition, we must know the "speed", i.e. the first partial derivatives of both of them;
- then we can calculate the second derivatives of both the metric tensor and the world lines of objects, and, accordingly, take the modeling step.
Here, however, lies one very unpleasant moment. It is not very simple to ask some initial state of the system: it can easily turn out that such a situation is simply unacceptable. There is no algorithm for constructing states - the sum of two valid states may turn out to be an invalid state. There are exactly four exact analytical solutions of the Hilbert-Einstein equations: the Schwarzschild, Kerr, Reisner-Nordstrom and Kerr-Newman metrics, which describe a space with one material rotating / non-rotating charged / uncharged point.
The numerical solution of the equations is also unlikely due to their tremendous complexity: for each point in four-dimensional space, you need to store four 16-digit matrices and solve 32 equations in each step, which is impossible at modern powers with any acceptable accuracy.
Finished with a general theory, let's move on to more private issues. Does GRT prohibit movement in decreasing time? Absolutely not. Aiming ds 2→ min, under some conditions, we can get dt <0 for the optimal trajectory of movement. Trying to formalize these “some conditions”, to put it mildly, is not easy; in particular, we can assume the existence of such a phenomenon as “wormholes” - special distortions of the geometry of space that allow traveling (possibly even safe for humans) at speeds faster than light (which is effectively a journey into the past - in a sense).
Does GRT clarify the nature of time? This is how to look. On the one hand, space, time, energy and momentum have acquired new meanings in the framework of the theory. On the other hand, questions to general relativity are formulated somehow not at all so simple and understandable as to Newtonian mechanics. For example, all GRT is covariant — it is written through tensors with lower indices (tensors with upper indices are called contravariant). Yes, I forgot to clarify - you just cannot change the position of the index :), the expression for changing from one coordinate system to another changes. So, why general relativity is covariant - one of the big philosophical questions, which is not something to answer, is not very easy to realize. Because.
If you look at modern, more "advanced" theories - string ones - then they are, in general, about the same. They only operate not with four-dimensional, but with 10- or even 11-dimensional space (in which all dimensions, except four, are “folded” so that they have zero size for us). In this space, multidimensional surfaces are considered (branes are multidimensional membranes) along which string objects move. The object moves so that its trajectory “sweeps” the minimum “area” on the surface of the “brane”. This is if you exaggerate. The meaning, in fact, is about the same, only there are more dimensions.
Here you can still tell about time in quantum mechanics - but thank you, this is already beyond the scope of my capabilities. There is almost nothing interesting in this place (quantum mechanics in this sense is more classical than GR - its laws are clearly written as time derivatives), except that the temporal coordinate of the object - like the spatial one - obeys the uncertainty relation and cannot be determined in pairs with energy is absolutely certain.
Now the time has come to return to the principle of causality. We see that GR does not give any insight in this place. Causation does not seem to exist. But you and I know perfectly well that it still exists, we very much see it in the world that is given to us in sensations.
GR (and string theories) are completely confused. If Newtonian mechanics clearly did not allow any such time travel, then GTR directly says: only the causality principle interferes with time travel and nothing more. Mathematically, nothing prohibits.
Hence, in order to get some kind of scientific answer to this question, one has to understand the meaning of the principle of causality. Alas, while in this place only fog of war is observed.
There is one theory that comes precisely from causality as the foundation of the universe. This is the so-called theory of causal dynamic triangulation, which builds the space of causal dependencies of events. (Again I exaggerate, google / Yandex in more detail.) Alas, this theory is not particularly remarkable, does not give any special insights - string theories so far look more interesting. However, some physicists have a different opinion - see Lee Smolin, "Problems with physics: the rise of string theory, the decline of science and what follows."
What is the result? As a result, I deceived you all. I’m going to be very surprised right away if it became even a little clearer to you how the time works and whether travel in it is possible; I myself got completely confused while writing this essay. I hope, on the other hand, that you now imagine the scale of the problem and realize that it is impossible to solve it all at once, fantasizing all sorts of nonsense :).
If Wikipedia is the main stronghold of truth and knowledge for you, and the physical laws are unshakable, then you should go to work in the Inquisition. So many more people who refute the theoretical inventions of physicists of the 18th century have not been burned!
I have not seen such a level of militant ignorance for a long time, and it’s doubly strange to see him on Habré.
Since I, unlike the author, is a scientist , I will try to tell the curious reader what we really know about the nature of time, causality and time travel.
Time
In classical mechanics, time is considered absolute and unchanged in the sense that during the transition from one reference system to another, time intervals do not change:
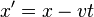



Here (x, y, z, t) are the coordinates in the old system, (x ', y', z ', t') - coordinates in the new system. Hereinafter, it is assumed (to simplify the formulas) that one system moves relative to another parallel to the x axis with speed v.
This is the so-called Galilean transformations - what happens to the coordinates when changing the frame of reference. In the Galilean sense, the “stream of time" is one throughout the Universe, and the time coordinates of all objects are the same. At the same time, classical mechanics does not in any way interpret the uniqueness of the arrow of time; Moreover, the very concept of the movement of time is not included in Newtonian formulas in any way.
In classical mechanics, we ourselves introduce a movement from the past to the future. Suppose we have a set of material points (coordinates and speeds) and acting forces. Next, we ask ourselves some dt interval and see how the system will evolve over time. No one is stopping us from moving in the opposite direction and keeping track of what happened to the system in the past.
However, "time travel" - i.e. the movement of one particular object on the t scale to the past is forbidden by Newtonian mechanics (see above - there is one time flow for the entire Universe).
The situation became much more fun when Maxwell formulated his electrodynamics, and then Einstein, in an attempt to solve the contradictions between electrodynamics and classical mechanics, created the theory of relativity.
Within the framework of the special theory of relativity in the transition from one inertial reference system to another, we use already just such transformations:
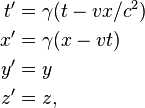 ,
, where
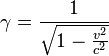 .
. Here, as is easy to see, each reference system has its own “time scale”. Within the framework of SRT, neither the spatial nor the time interval between points is preserved - only the difference of their squares is preserved:
ds 2 = c 2 dt 2 - dx 2 - dy 2 - dz 2 .
What is written here? This description of a special four-dimensional pseudo-Euclidean space - the so-called Minkowski space - in which the distance between the points is equal to the differencesquares of coordinate differences (and not the sum, as in ordinary Euclidean space).
From the point of view of SRT, the motion of each body is a trajectory in the four-dimensional Minkowski space - the so-called "World line".

The cones in the figure are the so-called “Light cones” are the trajectories of objects moving at the speed of light.
Since now the time of each object has its own - and, moreover, it is free to change as you like, we, theoretically, can allow for “reversals” of the world line, time travel.
(Traveling in time forward to the service station is very easy - just moving at a speed close to the light - and this effect, albeit on a microscopic scale, has been successfully confirmed. Therefore, we will hereinafter talk about time travel in the sense of time travelback )
However, there are two big problems.
The first is that a material object will never be able to reach the border of the light cone - this requires an infinite amount of energy to be applied.
The second is the curious features of the Lorentzian transformations. Suppose we were able to move an object from point A to point B faster than the speed of light. Then there is surely a reference system in which the object first appears at point B and then leaves from point A. Simply put, movement faster than light is a time movement in the SRT, but only in a very non-trivial sense.
Indeed, how do you understand this “there is such a system”? It turns out that for some observers there is a movement into the past, but for some it is not. It depends solely on the speed of movement of the observer.
And, secondly, it turns out complete madness with cause and effect. Obviously, the appearance of an object at point B is a consequence of its departure from point A. But for some observers, the effect occurs later than the cause. And for some - before. We will come back to this issue.
As a result, in the service station, movement faster than light is prohibited. No, more precisely, not so: the transfer of information between objects that are outside the light cone of each other is prohibited . Otherwise, the service station is faced with insoluble contradictions.
Initially, movement faster than light is allowed - provided that systems moving faster than light do not exchange information. For example, the inflation theory suggests that at the very beginning of the big bang, the family of newborn Universes was moving away from each other at a speed many times higher than light. And there is no contradiction here - because Universes move away from each other faster than light, light from one of them will never reach the other, and information will never be transmitted.
Let us return, however, to our sheep, i.e. to a discussion about the nature of time. To admit, STO has contributed quite a bit of understanding to this issue. What is the “internal time” of an object and how is it measured? Why is the time coordinate of Minkowski space so opposed to spatial? Finally, what kind of beast is such an “inertial reference frame” and where can it be taken in reality?
Einstein also understood all these difficulties, and therefore ten years later he created the general theory of relativity (GR), which completely confused everything.
So, for starters, all the coordinates in GR (including the time) are just the numbers of points on the axis. They have no more meaning, ask any.
Further, in these coordinates for each point in space-time it is necessary to determine the so-called fundamental metric tensor g ab . What kind of beast is this? This is a 4x4 matrix that determines the distance between adjacent points. Suppose you are at some point (x 0 , x 1 , x 2 , x 3 ) and are moving in the direction (dx 0 , dx 1 , dx 2 , dx 3 ). Then you will walk the distance:
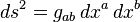
Hereinafter, summation is implied over the indices repeating from above and below, i.e. in fact, the sum of 16 components is written here.
For an ordinary Euclidean space with an orthonormal basis, the matrix g is equal to the identity. In Minkowski space, g 00 = 1, g 11 = g 22 = g 33 = -1.
In the space of general relativity, the components of the metric tensor can be absolutely anything. Those. the distance between adjacent points is an (almost) arbitrary function of the coordinate difference. Well, the coordinates are completely arbitrary, remember?
If suddenly we want to move from one coordinate system to another, all tensors (including the fundamental metric) will undergo these changes:
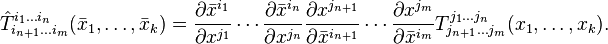
All in all, it was the easy part :). In short, in GTR you can ask for absolutely any coordinates and absolutely any reference point, the only thing you have to calculate is the fundamental metric tensor. The question about “how to measure the unique time of an object” does not seem to be worth it - number the moments of time as you like.
So, about the space-time geometry, it seems, as it turned out, we now turn to the objects in this space. From the distribution of momenta and body energies, we need to form the so-called energy-momentum tensor T ab . It is exactly the same at each point in space-time and sets the "density" of masses and energies.
Finally, we need derivatives of the fundamental tensor in all directions and derivatives (velocities) of all objects.
Now we can formulate how the geometry of space and the motion of bodies mutually influence each other.
The geometry of space changes as follows:
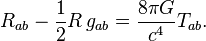
This equation is known as the Hilbert-Einstein equation. Here R ab - the so-called Ricci tensor expressed in terms of the second partial derivatives of the fundamental metric tensor. (By the way, Gregory Perelman casually proved Poincare’s theorem in his works devoted to the flows of the Ricci tensor.)
What is written here? Knowing the energy-momentum tensor, the metric tensor and the derivatives of the metric tensor, we can calculate the second derivatives of the metric tensor. Thus, we determine how energy and momentum affect the geometry of space.
Now back. To determine how the geometry of space affects the movement of objects in it, we direct for each material point the distance ds 2 → min passed by it . By revealing this condition (for example, through the Lagrange principle), we obtain expressions for the second derivatives (“accelerations”) of objects. In particular, for massless particles (light), movement occurs along a geodesic line expressed by the following equation:

Here Г a bc is the so-called. Christoffel symbols expressed through the metric tensor.
There is a rather elegant analogy that describes the meaning of such a movement in understandable terms. Imagine that we pulled a sheet and put heavy balls at some points. In these places, the sheet is bent and curved - just like space is curved in the presence of energy and momentum.
Now let's roll a light ball on our sheet. On flat areas, he will move in a straight line; but, approaching the massive points, he will bend his trajectory as if heavy balls "pulled" him. This is the effect of attraction, actually caused by the curvature of the “space” in which the ball moves.
Uhhhh Ok, to summarize how objects move in terms of GR:
- we must know the current geometry of space (metric tensor) and the position of the energies and momenta in it (energy-momentum tensor);
- in addition, we must know the "speed", i.e. the first partial derivatives of both of them;
- then we can calculate the second derivatives of both the metric tensor and the world lines of objects, and, accordingly, take the modeling step.
Here, however, lies one very unpleasant moment. It is not very simple to ask some initial state of the system: it can easily turn out that such a situation is simply unacceptable. There is no algorithm for constructing states - the sum of two valid states may turn out to be an invalid state. There are exactly four exact analytical solutions of the Hilbert-Einstein equations: the Schwarzschild, Kerr, Reisner-Nordstrom and Kerr-Newman metrics, which describe a space with one material rotating / non-rotating charged / uncharged point.
The numerical solution of the equations is also unlikely due to their tremendous complexity: for each point in four-dimensional space, you need to store four 16-digit matrices and solve 32 equations in each step, which is impossible at modern powers with any acceptable accuracy.
Finished with a general theory, let's move on to more private issues. Does GRT prohibit movement in decreasing time? Absolutely not. Aiming ds 2→ min, under some conditions, we can get dt <0 for the optimal trajectory of movement. Trying to formalize these “some conditions”, to put it mildly, is not easy; in particular, we can assume the existence of such a phenomenon as “wormholes” - special distortions of the geometry of space that allow traveling (possibly even safe for humans) at speeds faster than light (which is effectively a journey into the past - in a sense).
Does GRT clarify the nature of time? This is how to look. On the one hand, space, time, energy and momentum have acquired new meanings in the framework of the theory. On the other hand, questions to general relativity are formulated somehow not at all so simple and understandable as to Newtonian mechanics. For example, all GRT is covariant — it is written through tensors with lower indices (tensors with upper indices are called contravariant). Yes, I forgot to clarify - you just cannot change the position of the index :), the expression for changing from one coordinate system to another changes. So, why general relativity is covariant - one of the big philosophical questions, which is not something to answer, is not very easy to realize. Because.
If you look at modern, more "advanced" theories - string ones - then they are, in general, about the same. They only operate not with four-dimensional, but with 10- or even 11-dimensional space (in which all dimensions, except four, are “folded” so that they have zero size for us). In this space, multidimensional surfaces are considered (branes are multidimensional membranes) along which string objects move. The object moves so that its trajectory “sweeps” the minimum “area” on the surface of the “brane”. This is if you exaggerate. The meaning, in fact, is about the same, only there are more dimensions.
Here you can still tell about time in quantum mechanics - but thank you, this is already beyond the scope of my capabilities. There is almost nothing interesting in this place (quantum mechanics in this sense is more classical than GR - its laws are clearly written as time derivatives), except that the temporal coordinate of the object - like the spatial one - obeys the uncertainty relation and cannot be determined in pairs with energy is absolutely certain.
Now the time has come to return to the principle of causality. We see that GR does not give any insight in this place. Causation does not seem to exist. But you and I know perfectly well that it still exists, we very much see it in the world that is given to us in sensations.
GR (and string theories) are completely confused. If Newtonian mechanics clearly did not allow any such time travel, then GTR directly says: only the causality principle interferes with time travel and nothing more. Mathematically, nothing prohibits.
Hence, in order to get some kind of scientific answer to this question, one has to understand the meaning of the principle of causality. Alas, while in this place only fog of war is observed.
There is one theory that comes precisely from causality as the foundation of the universe. This is the so-called theory of causal dynamic triangulation, which builds the space of causal dependencies of events. (Again I exaggerate, google / Yandex in more detail.) Alas, this theory is not particularly remarkable, does not give any special insights - string theories so far look more interesting. However, some physicists have a different opinion - see Lee Smolin, "Problems with physics: the rise of string theory, the decline of science and what follows."
What is the result? As a result, I deceived you all. I’m going to be very surprised right away if it became even a little clearer to you how the time works and whether travel in it is possible; I myself got completely confused while writing this essay. I hope, on the other hand, that you now imagine the scale of the problem and realize that it is impossible to solve it all at once, fantasizing all sorts of nonsense :).
